INTRODUCTION
We have attempted in the preceding chapter to outline a system of historical geology which will explain all the actual data in a more comprehensive and consistent fashion than the evolutionary and uniformitarian framework which has been in vogue for the past hundred years. This proposed system finds its basic rationale in a frank recognition of the uniquely revelatory character of the Judaeo-Christian Scriptures. Beginning with the realization that a uniformitarianism based on present processes not only has not but cannot provide a scientifically correct explanation of early geophysical and biologic history, we recognize that any genuine knowledge of these matters must necessarily come by way of some form of divine revelation.
The unique claim of the Bible, supported by the testimony of Jesus Christ Himself, and of nineteen hundred years of Christian history, that it embodies this revelation is more than adequate warrant for us to base a proposed framework for geologic history on the facts recorded therein. Accordingly, an attempt has been made to determine how the actual data of geology and paleontology can be understood in full harmony with these revealed facts, especially with the fact of a genuine Creation and the fact of a great world-destroying Deluge. We submit that the data, at least in broad outline as presented in the preceding chapter, have been shown thus to harmonize quite remarkably with the Biblical record. Such a demonstrated harmony does not of course indicate any particular insight or originality possessed by the writers but only gives testimony to the veracity and perspicuity of the inspired accounts in the Bible.
It is certainly recognized that not all questions have been answered or all problems resolved. A complete reorientation of all the enormous accumulations of pertinent data and interpretations would take not a few hundred pages, but several large volumes at least, and would require the intensive efforts of a great number of specialists trained in the various areas of geology and geophysics. But the Biblical framework can at least point the way for such studies, and it provides the basic key with which all such problems can be ultimately resolved.
This chapter can only deal with some of the major aspects of some of the major problems. But if it has indeed been shown that the general features of the geological data all harmonize with the Biblical outline, and if it can now be shown that the major apparent difficulties in this system can likewise be resolved and understood in these terms, then it is reasonable to conclude that the smaller problems can also be eventually solved by further study.
METHODS AND RESULTS OF GEOCHRONOLOGY
By all odds, the most important and serious of these problems is that of time. There are many lines of geological evidence apparently implying that the earth and its various crustal formations are immensely older than a straightforward Biblical system of interpretation can allow. The latter, as we have seen, involves a relatively recent Creation and Deluge as the cause of most of the earth's geologic features.
There are many different ways by which geologists have attempted to measure the absolute age of the earth and its various formations and deposits. In each such method, some physical or chemical process is found whose present rate of activity can be measured. The total accumulation of the product of the process must also be measured. It is a simple matter of mathematics then to calculate how long the process must have been in operation in order to have produced its present results. Some of the processes which have been used as supposed geologic chronometers involve the influx of sodium and other chemicals into the ocean and into lakes from rivers, the erosion of gorges or other areas by running water or wind or glaciers, the building of deltas or other sedimentary deposits, the growth of chemical deposits in soils or caves or other places, the weathering of rocks, the accumulation of annual bands in trees or lake beds or other entities whose appearance may be affected by seasonal changes, the escape of terrestrial gases into the atmosphere, the efflux of connate waters through volcanism to the earth’s surface, and various other like processes. There are also various astronomic chronometers that have been used to determine absolute age, most of them based on the rate of expansion of the universe and its various component parts and on the velocity of the light coming from distant galaxies. The most important geologic chronometers are of course those based on the phenomenon of radioactivity. Various chemical elements are in some degree radioactive, disintegrating continuously into another element or isotope. The rate of disintegration can be measured and if a mineral containing measurable quantities of both the parent and daughter elements is found and analyzed, then a relatively simple mathematical computation will yield the time period during which the daughter element has apparently been accumulating by the process. The most important of these radioactivity methods involve the disintegration of uranium and thorium into radium, helium, and lead; of rubidium into strontium, and of potassium into argon and calcium. Of a somewhat different type is the radiocarbon method, based on the formation of radioactive elements of carbon in the atmosphere by cosmic radiation and their subsequent decay to the stable carbon isotope.
There is no question that the vast majority of these geochronometers have given estimates of geologic age immensely greater than any possible estimate based on Biblical chronology. The radioactivity estimates, in particular, (except the radiocarbon method) usually yield age values measured in hundreds of millions of years and some up to three billions of years.
But the accuracy and significance of any or all such measurements are of course based entirely upon the accuracy with which the measurements can be made and the assumptions which enter into their interpretation. Far too little account has been taken of the limitations which these factors impose.
THE LEAD AGE METHODS
Consider, for example, the various methods based on disintegration of uranium and thorium into lead. Each of the parent elements disintegrates by some process through a certain chain of elements and isotopes until it reaches a stable condition. Geochronological use of these facts requires very accurate measurements of the amounts of the various elements of the chain present in the mineral and also very accurate knowledge of the respective decay constants. The techniques for these determinations are exceedingly difficult and subject to large error.
Although radioactivity measurements of geologic age have been widely accepted for some fifty years and have been responsible for the wide acceptance of an age for the earth measured in billions of years, it is now generally admitted that most of the work done before 1950 was quite misleading, mainly because of defective measurements or interpretations of the measurements. One of the main workers in this field, Dr. L. T. Aldrich, says:
Between this classic pioneer work (i.e., the discovery of the several uranium and lead isotopes about 1930) and 1950, only a handful of mineral ages were accurately determined. The reason for this was primarily the requirements that the mineral contain one percent or more of uranium and/or thorium, so that the chemical determinations of these two elements and the daughter element, lead, could be made by the standard techniques of analytic chemistry. Even for such minerals, serious errors of analysis were very common.1
1 L. T. Aldrich, “Measurement of Radioactive Ages of Rocks,” Science, Vol. 123, May 18, 1956, p. 871.
Partly because of the inadequacies of the measurements, most of the ages published in the literature were discordant and therefore rejected.
It was found during this pioneering period that the three ages derived from the radioactive series of uranium and thorium on the same mineral were often discordant, and in fact the geologic time scale given by Holmes [i.e., by Arthur Holmes, the leader in the development and popularization of the radioactivity methods] is based in part on discordant ages which are very difficult to interpret unambiguously.2
2Ibid. Gordon Gastil has recently reminded his colleagues: “Attempts to measure mineral age began soon after the discovery of natural fission. During each decade since then, analysts have discarded most of the age determinations made in the preceding one.” (“The Distribution of Mineral Dates in Time and Space,” American Journal of Science, Vol. 258, Jan. 1960, p. 4).
A more important reason for the errors in the earlier published ages was the neglect of the factor of original lead in the mineral. Obviously, if some of the lead in the sample was non-radiogenic, then the computed age would be too large by an indefinite amount, unless the “common” lead were first determined and eliminated from the calculation. This is verified by Knopf:
The contaminating lead would make the calculated age too great, and it must be allowed for. To make the proper correction, especially if the correction is a considerable one, an isotopic analysis of the common lead that had been deposited in the same district and at the same time as the radioactive mineral must be used. The necessity for this rigorous requirement has only been recognized within the past several years.1
1 Adolph Knopf, “Measuring Geologic Time,” Scientific Monthly, November 1957, Vol. 85, p. 230.
Since measurement techniques have been highly refined in recent years and since common lead corrections on the above basis are now made in most computations,2 these criticisms are not particularly serious at present. But it is well to be reminded of the history of the radioactivity method. Its proponents 20 and 30 years ago were maintaining its finality and the validity of its estimates of absolute time as dogmatically as do its present-day expositors, even though the vast majority of their calculations are now known to have been quite wrong. It may be that currently accepted results will one day have to be rejected as well, for reasons yet unrecognized.
2 However, recognition of supposed common lead contamination depends on detection of lead of atomic weight 204 in the mineral. Ore lead contains a small amount of this isotope along with larger but varying amounts of 206, 207, and 208 atomic weights. Each of the latter isotopes can also be produced radiogenically. However, the all-important amount of 204 lead is quite difficult to determine accurately. As G. R. Tilton points out: “It should be realized that the Pb 204 abundance is the least accurately known of all the isotopic abundances for the leads” (“Interpretation of Lead-Age Discrepancies,” Transactions, American Geophysical Union, Vol. 37, April 1956, p. 225.)
Other possible sources of error are known to exist, of course, and have often been used as the basis for rejecting measurements which seemed incapable of harmony with the accepted chronology. Hahn indicates one possibility:
It may be that part of the lead was leached out; then the age determined would be too low. However, it is also possible that uranium was removed; then relatively too much lead would be found, and the age determined would be too high. It follows that reliable lead values can be expected only from specially selected, dense mineral samples that are weathered as little as possible.1
1 Otto Hahn, “Radioactive Methods for Geologic and Biologic Age Déterminations,” Scientific Monthly, Vol. 82, May 1956, p. 258.
The serious probability of significant uranium leakage is clearly shown by the following:
Most igneous rocks also contain uranium in a form that is readily soluble in weak acids. Hurley (1950) found that as much as 90 percent of the total radioactive elements of some granites could be removed by leaching the granulated rock with weak acid. . . . Larsen and Phair (in Faul, 1954, p. 80) note that ‘commonly, as much as 40 percent of the uranium in most fresh-appearing igneous rocks is readily leachable.’ ”2
2 M. R. Klepper and D. G. Wyant, Notes on the Geology of Uranium, U. S. Geological Survey Bulletin 1046-F, 1957, p. 93.
The seriousness of these defects is also pointed out by Faul:
Countless determinations have been made by this method, but it was found that the premises on which the method rests are not valid for most uranium minerals. There is definite evidence of selective uranium leaching by acid waters, and it is now known that most radioactive minerals contained some lead when they were formed. As a result, most of the early lead :uranium age determinations are questionable.3
3 Henry Faul, Nuclear Geology (New York, John Wiley & Sons, 1954), p. 282.
Several auxiliary methods have been devised with the uranium series, in order to attempt to obviate some of these difficulties. Each involves ratios of two of the elements in the disintegration series. Each seems to have certain advantages and applications, but each also has quite definite disadvantages. For example, concerning the series which proceeds from the uranium 238 isotope to the lead 206 isotope (the numbers refer to atomic weights). Faul says:
The chief disadvantages are that hexavalent uranium is readily leached and that radon 222, which forms in the decay of uranium 238, has a half-life of 3.82 days and, being gaseous, might escape from the system.1
1 Faut, op. cit., p. 294.
Uranium 235 decays through a different series to lead 207 but is present in such infinitesimal amounts as to curtail its usefulness seriously. It also is subject to uranium leaching, though not so much to radon leakage. Both methods are also subject to lead enrichment or removal during geologic time.
Deficiency of lead could be due to the loss of lead itself or escape of some intermediate member of a decay chain. ... no satisfactory solution to the actual cause of apparent lead deficiency has yet been found. . . .2 It may be noted in passing that these apparent lead deficiencies found in so many minerals have been adjudged deficient primarily because the calculated ages turned out to be discordantly low.
2 L. H. Ahrens: “Radioactive Methods for Determining Geologic Age,” in Physics and Chemistry of the Earth, ed. by Ahrens, Rankama, & Runcorn, (New York, MeGraw-Hill, 1956, pp. 49-50).
Another method consists of comparing the relative amounts of the two lead isotopes, 206 and 207, which are present in the mineral, since these have been produced at different rates through different decay chains. This method has in recent years been considered as one of the most reliable. But:
Actually, the method is subject to several errors. Loss of radon 222 raises the lead :lead ratio and the calculated age. A rather large error may be introduced by the uncertainty in the composition of the original lead. This error may exceed the measured value when dealing with younger uranium minerals containing even small amounts of original lead, as clearly recognized by Holmes when the method was first proposed. Presence of old radiogenic lead (formed in a prior site of the parent uranium) may cause great error. Instrumental errors in mass spectrometry may yield consistently high apparent proportions of lead 204 and lead 207. Redistribution of elements by renewed hydrothermal activity may be a serious source of error in all lead methods.3
3 Faul, op. cit., p. 295.
One of the above-named sources of error may be particularly significant. Although it is common now to attempt to allow for contamination by original common lead by assuming that the presence of lead 204 in the mineral indicates such contamination, it appears also quite possible that many, or most, such minerals might equally well contain some contaminating radiogenic lead from some other source; if so, the age computation would of course be too high by a quite unknown amount. The possibility of this type of phenomenon occurring is indicated by a recent study at the University of Toronto:
There are some leads that have been referred to as anomalous which have isotope ratios that do not, at first sight, seem to participate in this regularity. We believe that additional amounts of radiogenic lead have been added to these leads, at or about the time of final mineralization. That is, an anomalous lead is simply an ordinary or non-anomalous lead which has been further altered.1
1 R. M. Farquhar and R. D. Russell: “Anomalous Leads from the Upper Great I akes Region of Ontario," Transactions, American Geophysical Union, Vol. 38, August 1957, p. 552.
The above authors were concerned about the fact that too much radiogenic lead was present in certain supposedly ancient lead ores to harmonize with the theory that “common” lead has been uniformly enriched during geologic time with increments of radiogenic lead as evidenced by the larger proportion of lead isotope 204 in older common leads. These anomalous leads show less 204 lead than should be present according to the theory. The really significant thing, however, is that it is thereby evident that radiogenic lead can contaminate any uranium-lead bearing mineral to an unknown amount and thereby make any age determination on it meaningless.
That such contamination of ordinary lead deposits by radiogenic lead is far from rare is indicated by the following:
True ordinary leads are probably derived from below the crust, and anomalous leads are derived in turn from these by variable radiogenic con-lamination in the crust. Thus ordinary and anomalous leads form a series rather than two distinct groups. It is likely, furthermore, that no absolutely ordinary leads occur on the earth’s surface, as all have probably received at least minute radiogenic contamination in coming from the mantle.2
2R. L. Stanton and R. D. Russell: “Anomalous Leads and the Emplacement of Lead Sulfide Ores" Economic Geology, Vol. 54, June-July 1959, p. 606.
Thus, as Boyle recognizes:
The ratio of the lead isotopes in deriving their lead from such rocks is, therefore, neither a measure of the age of the deposits nor the age of the sedimentary host rocks but is rather a function of the complex geochemical processes through which the lead may have passed.1
1 R. W, Boyle: “Some Geochemical Considerations on Lead Isotope Dating 0£ Lead Deposits,” Economic Geology, Vol. 54, Jan-Feb. 1959, p. 133.
In spite of the necessarily unknown amount of radiogenic contamination of all lead deposits, the theory that common leads have been uniformly enriched by gradual accumulations of radiogenic lead during geologic time has been made the basis of probably the most important present geologic estimate of the total age of the earth’s crust, leading to a figure of the order of five billion years. As Harrison Brown claims:
Thus solely on the basis of the isotopic composition of common leads we can say that the age of the earth probably lies somewhere between 3.1 and 5.6 billion years.2
2 Harrison Brown: “The Age of the Solar System,” Scientific American Vol. 196, April 1957, p. 86.
This sort of calculation, though containing numerous unverifiable assumptions, has been widely accepted and circulated, but there are many who remain unconvinced. After a rather lengthy and impelling criticism of the method, especially on the basis of its very subtle and speculative assumptions, a triad of authors (one from California Institute of Technology, one from Carnegie Institute in Washington, one from Chicago University), concludes:
In view of the evidence for extensive mixing, it would seem contrary to the facts to postulate differing frozen lead-uranium ratios that have existed for billions of years. The requirements of the assumptions in the ore lead method are so extreme it is unlikely that it should give a correct age.3
3C. Patterson, G. Tilton, and M. Inghram. “Age of the Earth.” Science, Vol. 121, January 21, 1955, p. 74.
It would seem, therefore, that it is quite possible for any lead deposit or any mineral containing lead (including the uranium minerals on which most age-estimates have been based) to contain substantial though unknown amounts of antecedent radiogenic lead. This would necessarily make all such age-estimates too high by an unknown amount.
From these examples it is readily apparent that the amount of accumulated radiogenic lead contributed to a deposit is the deciding factor in age determinations and must be known before any age can be assigned to a deposit.1
1 Boyle, op. cit., p. 135.
Still other methods have been used to some extent, for example, the thorium-lead 208 ratio. As Aldrich says, however:
The two uranium-lead ages often differ from each other markedly, and the thorium-lead age on the same mineral is almost always drastically lower than either of the others.2
2 L. T. Aldrich: “Measurement of Radioactive Ages of Rocks,” Science, Vol. 123, May 18, 1956, p. 872.
Apparently a satisfactory explanation of this conflict is not yet available:
Most of the ages obtained by the lead :thorium method disagree with the ages of the same minerals computed by other lead methods. The reasons for this disagreement are largely unknown.3
3 Henry Faul: Nuclear Geology (New York, John Wiley & Sons, 1954, p. 295.)
Another method is the lead 210 method, lead 210 being a particular stage in the decay series leading to lead 206. The ratio of lead 206 to lead 210 is used to compute the age of the mineral. But as Faul says:
Unfortunately, the lead 210 method is subject to similar errors as the lead :uranium and lead :lead methods, owing to loss of constituents of the radioactive series of leaching or emanation.4
4 Ibid.
The very light gas, helium, is a product of the disintegration of uranium and thorium, along with lead, and helium measurements in minerals have long been used as indices of age. The method has had many ups and downs in the favor of geophysicists, due to expertmental difficulties and the presumed ease of helium leakage. In a recent review of the present status of all the various radioactivity methods, Dr. Adolph Knopf concludes:
Because of such uncertainties about the helium age determinations, the method has again fallen into nearly complete disuse.5
5 Adolph Knopf: “Measuring Geologic Time,” Scientific Monthly, Vol. 85, Nov. 1957, p. 228.
After listing all the various requirements for successful determination of an age by the lead method, Rankama says:
No radioactive minerals have been analyzed that satisfy all these requirements. Consequently, errors are liable to creep into the calculated lead ages. In particular, the alteration of radioactive minerals is the cause of errors in the age values. Even the freshest-looking minerals usually have gained or lost small quantities of the pertinent nuclides.1
1 Kalervo Rankama: Isolope Geology (New York, McGraw-Hill, 1954), p. 379.
In view of all the sources of error in the various uranium-thorium series methods, it is small wonder that most age measurements have been found hopelessly discrepant and have been rejected. Only those few minerals which give agreement by more than one method are now considered really reliable, and these are so few and far between that at least some of these apparent agreements can be explained on the basis of pure chance.
It appears that the best criterion for a reliable age determination is the agreement of age values calculated from the lead 207-lead 206, lead 206-uranium 238, and lead 207-uranium 235 ratios, even though the lead 208-thorium 232 age may be discordant. This happy situation occurs in the case of some pegmatitic radioactive minerals, and in the case of a few pitchblendes, but seems to be the exception rather than the rule.2
2 National Research Council: “Report of the Committee on the Measurement of Geologic Time,” 1957, p. 4.
In addition to all the difficulties encountered in these methods, they have been of limited usefulness because of the extreme rarity of uranium and thorium minerals, especially in fossiliferous rocks. Consequently, much attention has been given in the past decade to the development of methods involving the radioactive isotopes of the alkali metals, rubidium and potassium. These are much more common, and the potassium minerals especially are commonly found in sedimentary rocks.
One of the main workers in the development of the rubidium-strontium method has been Dr. Otto Hahn. The main question about the method has been the lack of agreement concerning the disintegration rate of rubidium. Hahn says:
For this method, however, a knowledge of the transformation rate of rubidium into strontium is necessary. The final decision regarding the half-life has yet to be made.1
1 Otto Hahn: “Radioactive Methods,” Scientific Monthly, Vol. 82, May 1956, p. 261.
Ahrens, another leading worker in this field, gives a list of different determinations of the half-life of rubidium as made by various scientists, showing a variation all the way from 48 to 120 billion years.2 A further limitation is the very small amount of strontium present and the fact that much of this may be non-radiogenic.3
2 L. H. Ahrens: Physics & Chemistry of the Earth (New York, McGraw-Hill, 1956, p. 54.)
3 Hahn, op. cit., p. 262.
Potassium was proved about ten years ago to decay by two different processes into calcium and the gas argon. Because of the wide incidence of potassium minerals in sedimentary rocks, this has seemed to be a potentially very fruitful geochronologic device. Again, there are serious difficulties, however. As Wetherill says:
The two principal problems have been the uncertainties in the radioactive decay constants of potassium and in the ability of minerals to retain the argon produced by this decay.4
4 G. W. Wetherill: "Radioactivity of Potassium and Geologic Time,” Science, Vol. 126, September 20, 1957, p. 545.
Although the decay rates are still a matter of considerable uncertainty, the more serious problem is that of argon loss. Potassium is found mainly in feldspars and micas, and it is believed, on the basis of comparative age measurements with other methods, that the feldspars in general must have lost about half of their radiogenic argon through emanation from the mineral. It is maintained, however, that the micas in general are able to retain most of the argon. But again Wetherill admits:
In view of the fact that fairly low retentivities sometimes occur even in the case of mica, measurement of the potassium-argon age of a mica does not give a completely trustworthy value of the age.5
5 Ibid., p. 549.
Thus, as we examine one by one the various radioactivity methods for measuring geologic age we find that each encounters many serious problems in it use — enough to cast grave doubt on the reliability of any age computed from it. The potassium-calcium method is even less reliable than the potassium-argon method, owing to the fact that radiogenic calcium (of atomic weight 40) is impossible to distinguish from other calcium 40 which is commonly found present in potassium minerals. Hahn says:
Unfortunately, calcium 40 is the most frequent partner of the regular mixed element calcium. Therefore, only in very old potassium minerals, nearly completely free of calcium, is it possible to find through extremely accurate mass spectroscopy the very small shift in the isotope ratio of calcium, and thus use the activity of the potassium for age determination.1
1Otto Hahn, op. cit., p. 261.
THE SIGNIFICANCE OF THE RADIOACTIVITY DATA
It thus becomes evident that age measurements by radioactivity are not nearly so precise nor so reliable as most writers imply. The great variety of possible experimental errors and physical alterations in the quantities being measured have all combined to produce such a high degree of statistical scatter in the results of the computations, especially when compared with the geochronological implications of the associated stratigraphy, that the great majority of the measurements have had to be rejected as useless for the desired purpose. Relatively, only a handful has been acceptable.
But of course it will be answered that, even though experimental errors may be important, the measurements are still sufficiently accurate to give in most cases ages of at least the right order of magnitude. For example, a measurement indicating an age, say of one billion years, could hardly be in error by more than a factor of 10, and this would still give a hundred million years, nothing remotely comparable to the few thousand years implied by the Bible. Furthermore, it will be maintained that even though any given age measurement may be completely erroneous due to leaching or emanation or some other effect, there are many cases now known where the age estimate has been checked by two or more different methods, independently. It would seem improbable that the elements concerned would have each been altered in such a way as to continue to give equal ages; therefore, such agreement between independent measurements would seem to be strong evidence that alteration had not occurred and that the indicated age is therefore valid.
We reply, however, that the Biblical outline of earth history, with the geologic framework provided thereby, would lead us to postulate exactly this state of the radioactivity evidence! We would expect radiogenic minerals to indicate very large ages and we would expect different elements in the same mineral, or different minerals in the same formation, to agree with each other! The fact that so many calculations fail to agree or to fall into proper place in the stratigraphic sequence is strong testimony that uniform processes do not constitute the norm in earth history. The great number of “discordant ages,” of “anomalous leads,” and the like, testify to the intense mixing activity of the Deluge and other catastrophic geologic events.
This may appear to many to be a surprising assertion, but a little consideration should suffice to show its validity. The whole problem revolves about the basic assumptions implicit in all the radioactivity methods of measurement. In addition to the problems of measurement and alteration already discussed, there are two basic assumptions always present. One is that all of the identified radiogenic isotope has been derived from the parent isotope by radioactive disintegration. The other is that the rate of disintegration has always been the same as at present. Both these assumptions are absolutely necessary in order to obtain any kind of meaningful age measurement. But neither assumption can possibly be valid if the Bible account is true! They implicitly deny the two divinely revealed facts of a genuine Creation and at least one great discontinuity in the uniform processes of nature at the time of the Deluge.
THE FACT OF A “GROWN” CREATION AND “APPARENT AGE”
We have already shown1 that the Bible quite plainly and irrefutably teaches the fact of a “grown” Creation — one with an “apparent age” of some sort, analogous to the “apparent age” of a mature Adam at the first instant of his existence.2 This Creation must have included all the chemical elements already organized in all the organic and inorganic chemical compounds and mixtures necessary to support the processes of the earth and of life on the earth. These processes include the phenomena of radioactivity. It is perhaps possible that only the parent elements of the radioactive decay chains were originally created, but it is eminently more harmonious with the whole concept of a complete Creation to say that all the elements of the chain were also created simultaneously, most likely in a state of radioactive equilibrium.
1See page 218-19, 223-24, and 232-35.
2The uniqueness of Adam and Eve’s creation (see also p. 456) is emphasized in the New Testament: “For Adam was first formed, then Eve” (I Tim. 2:13), "... for the man is not of the woman, but the woman of the man" (I Cor. 11:8). Similarly, most of the Biblical miracles stress true creative activity, in which the time factor is immensely compressed: for example, the transformation of water into wine (John 2:10), creation of "apparent age” in other words.
This means that, with each mineral containing a radioactive element, there were also at the original Creation all of the daughter elements in the decay series, including some of the final stable end-product. Such a concept is undoubtedly shocking to the mind of a consistent uniformitarian, but there is nothing impossible or unreasonable about it. In fact, short of denying the existence of any Creator or original Creation at all, one must logically come to some place in the long chain of secondary causes where something was created. If so, that something, at the instant of its creation, must have had an “appearance of age.” And the only way we could then determine its “true age” would be through divine revelation. An “apparent age” might of course be deduced for that something on the basis of any processes of change which were observed in connection with it, but this would not be the true age.
And this is exactly the situation we find in connection with these radioactive elements and with many other geochronometers. It is eminently reasonable and consistent with the basically efficient and beneficent character of God, as well as with His revelation concerning the fact, that He would have created the entire universe as a complete, operational, functioning mechanism. The grossly cruel and wasteful processes of an almost interminable evolution leading up to man’s arrival as its goal, as usually envisioned by uniformitarians, (or at least by theistic uniformitarians), are on the other hand utterly inconsistent with the character and wisdom of God! It is therefore not ridiculous after all, but perfectly reasonable, to suppose that the radiogenic elements, like all other elements, were created directly by God.
The obvious question then arises as to whether the “apparent ages of the minerals so created, as indicated by the relative amounts of “parent” and “daughter” elements contained therein, would all be diverse from each other or whether they would all exhibit some consistent value; and if the latter, what value of apparent age might be implied.
In the absence of specific revelation, it seems impossible to decide this question with finality. However, it is more satisfying teleologically, and therefore more reasonable, to infer that all these primeval clocks, since they were “wound up” at the same time, were also set to “read” the same time. Whatever this “setting” was,1 we may call it the “apparent age” of the earth, but the “true age” of the earth can only be known by means of divine revelation.
1 It is interesting to note that Peter, when discussing the duration of terrestrial history, emphasizes as significant the fact that: “One day is with the Lord as a thousand years” (II. Peter 3:8), thus stressing the time-transcendent nature of God. Perhaps God has also emphasized this truth in His physical creation, by means of “setting the clocks” of natural processes to read such tremendous ages as they appear to do. Yet the Biblical revelation of actual human and earthly history indicates a relatively ephemeral existence, beginning only some eight to ten thousand years ago.
VARIATIONS IN THE DECAY RATES
But this is not the only assumption in age calculations. Regardless of whether or not the original mineral was “set” to read a certain finite time at the instant of its creation, we still could not know for certainty what this original condition had been, since we cannot know to what extent the rate of decay has varied since that time.
It is possible, of course, to measure or estimate the decay rates as they exist now for each of the radioactive series and for each stage in the series, and this has been done. As we have seen, considerable question still exists as to the proper value for many of these decay constants, but the values of all the important ones are known to at least the right order of magnitude. And of course the claim is made that these decay rates never change and that it is, therefore, legitimate to use them in the computation of ages. Extremes of temperature, pressure, physical state, chemical combination, etc. have been applied to the radioactive elements without any significant indication of resulting changes in the disintegration constants. It is claimed that no past change in terrestrial environments, as conceived accord ing to uniformitarian principles, could have been outside the scope of these laboratory studies. It is, therefore, maintained that the decay rates have never changed.
There is nothing basically inviolable about these decay rates, however. This is proved by the fact that it has been found possible to change some of them at least slightly, in the laboratories.
Experiments with decay of two artificial isotopes thought to be the most sensitive to change in atomic structure (beryllium 7 and an excited state of technetium 99) have shown that the decay rate can be changed, but the change is extremely small.1
1 Henry Faul: Nuclear Geology, p. 10.
These changes were due to changes in the chemical compounds of which the elements were a part, but similar small changes in certain decay rates can be effected by pressure.2
2 Ibid.
There are several types of radioactive disintegration that are known to occur in nature. Alpha-decay consists of the emission of nuclei of atoms of helium 4 from nuclei of heavy atomic weight. This is the type of decay initiating the uranium and thorium series, whose disintegration results finally in lead and helium with several intermediate elements in the chain. Beta-decay consists of the emission from the nucleus of a beta-particle (an electron) and a neutrino; this is the decay process involved in the formation of strontium 87 from rubidium 87 and of calcium 40 from potassium 40. A third type of decay is the capture of an orbital electron by the nucleus, accompanied by the emission of X-rays. The formation of argon 40 from potassium 40 is of this kind. A fourth kind of decay is nuclear fission, by which the nucleus splits into two discrete parts. This is the action of the atomic bomb, but it also occurs in nature. The uranium 235 isotope is subject to fission by free neutrons in the earth, from whatever source. Uranium 238 and thorium 232 undergo a process of spontaneous fission, whereby occasional atoms, under the pressure of high internal proton charge, spontaneously break into two parts. In this process, the main products are the rare gases xenon and krypton, along with neutrons and other particles.
Each of these processes is interpreted essentially as a statistical process, with the particular rate of decay being a probability function related to the type of process and the element concerned. Each is known to be related to the structure of the atomic nucleus and the various nuclear forces and particles. But, although the intensive research devoted to modern nuclear physics has yielded a tremendous amount of information about the various nuclear particles and reactions, most of these formulations are still largely empirical, with very little basic understanding of why the nucleus behaves as it does. As Beard has said:
We comprehend quite well what nuclear structure is; but as yet we are only beginning to see why it is.1
1 David B. Beard: “The Atomic Nucleus,” American Scientist, Vol. 45, Sept. 1957, p. 342.
Similarly, George Gamow, who has made many significant contributions to nuclear physics, including in particular the present interpretation of the alpha-decay process, in a recent review points out:
Although experimental studies of these new particles reveal new and exciting facts about them almost every month, theoretical progress in understanding their properties is almost at a standstill.2
2 George Gamow: “The Exclusion Principle," Scientific American, Vol. 201, July 1959. p. 86.
Alpha-Decay and the Potential Barrier
With respect to the alpha-decay process, which is the most important process from the standpoint of geologic time measurement, the best theoretical explanation developed to date is Gamow’s suggestion, formulated in terms of wave mechanics and statistical probabilities. According to this concept, although the energy of the alpha-particle is apparently too small to permit it to escape from the “nuclear potential barrier” of energy surrounding the nucleus, nevertheless it has a certain small probability of doing so.
According to classical mechanics, the incoming or outgoing nuclear particles can pass the potential barrier only if their kinetic energy is larger than the maximum height of the barrier. Experimental evidence shows, however, that this is definitely not so. An example is represented by a uranium nucleus, which has a radius of 9 x 10 13 cm. and is surrounded by a potential barrier 27 Mev high. Since the alpha-particles that escape from uranium in the process of its natural decay have an energy of only 4 Mev, it is difficult to understand how they get out across the barrier at all. ... It turns out, in fact, that the wave mechanics of a particle permit it to do things that would be completely prohibited in classical mechanics. . . . Using wave mechanics, we can calculate that the chances of getting through are about 1 in 1038.1
1George Gamow: Mauer, Earth, and Sky (Englewood Cliffs, N. J., Prentice-Hall, Inc., 1958), pp. 341-342.
The symbol Mev stands for a “million electron volts,” an electron-volt being the energy imparted to a single electron when it is accelerated by a one-volt electric potential. (Similarly, Kev stands for “thousand electron-volts, Bev for billion electron-volts, etc.). The probability of escape of an alpha-particle through the energy barrier erected by the high nuclear forces in the atom depends on the relation between the energy of the particles and that of the barrier, and these factors vary in some incompletely-understood manner from one nuclear species to another. The nearer the energy of the alpha-par-tides to that of the barrier, the more probable is the escape of any single particle, and, therefore, the more rapid the general decay of the nucleus. Thus, the “decay constant” of any given radioactive element depends on the relative energies contained in its nucleus.2
2Thus: “In general, it can be said that this probability is greater, the larger the energy of the alpha particle relative to the top of the barrier, and the smaller the “thickness” of the barrier at the point corresponding to the given energy value. . . . It follows, therefore, that the greater the energy of the alpha particle in a radioactive atom, the more likely is it to be found outside the nucleus” (Samuel Glasstone: Sourcebook on Atomic Energy, 2nd Ed., New York, D. Van Nostrand & Co., 1958, pp. 173-174).
Herein lies the reason for the apparent constancy of these decay rates. The energies are so high that any ordinary external energy source, whether physical or chemical, is of entirely too low an order of magnitude to have any effect.
After Rutherford became completely persuaded that the radioactive decay of heavy elements is due to the intrinsic instability of their atomic nuclei, his thought turned to the possibility of producing the artificial decay of lighter and normally stable nuclei by subjecting them to strong external forces. True enough, it was well known at that time that the rates of radioactive decay are not influenced at all by high temperatures or by chemical interactions, but this could be simply because the energies involved in thermal and chemical phenomena are much too small as compared with the energies in the nuclear disintegration phenomena.1
1 George Gamow: Matter, Earth, and Sky, 1958, p. 330.
Rutherford proceeded to bombard his nuclei with high-energy alpha-particles, and the whole subsequent history of nuclear physics has demonstrated the possibility of penetrating the nucleus, through the potential barrier, provided only that a source of sufficiently high energy is used.
It is, therefore, evident that the basic decay relationships could be changed if something were done to change the relationship between the energy of the alpha-particles in the nucleus and the nuclear forces creating the potential barrier. Although the exact nature of these forces is still uncertain, it seems evident that some external source of sufficiently high energy level would be required. Pressures, temperatures, chemical reactions, ordinary radiations are all inadequate, and therefore the decay rates seem to be constant. Nevertheless, if an environment of high-energy radiation could be imposed on the elements, it seems certain that the balances, and therefore the decay phenomena, would be altered.
Such an environment may be difficult, or impossible, to impose in the laboratory, and in any case it supposedly could not have been produced at any time in the earth’s past history as a geologic environment and so could have had no influence on the decay constants.
But this is an entirely gratuitous assumption. Such an environment does exist, right now, in the earth’s upper atmosphere, where a great variety of radiations, including particles of fantastically high energies, exist in profusion. If any very substantial part of this radiation has ever in the past been able to penetrate to the lower atmosphere and into the earth’s crust, it must have had some substantial effect on the radioactive decay rates of the unstable atomic nuclei. And, in view of the Biblical record of the Creation and the Flood, it seems likely that a large amount of this radiation may have reached the earth’s surface during the creation before the establishment of the earth’s thermal vapor blanket and during the Flood, immediately after its dissipation and before the development of the present atmospheric regime.
Of particular interest in this connection are the intensely powerful cosmic rays. The character of these rays is indicated by the following:
To begin with, primary cosmic radiation, that is, the rays as they exist in space, is composed of atomic nuclei traveling with speeds so enormous as to approach that of light (186,000 miles per second).1
1 Arthur Beiser: “Where Do Cosmic Rays Come From?”, Scientific Monthly, Vol. 77, August 1953, p. 76.
The rays are mainly nuclei of atoms, of many of the chemical elements, especially hydrogen and helium but also including heavier elements. The energies of these particles are tremendous, ranging from one billion to over a billion billion electron volts, far beyond the capacities of our largest man-made accelerators (compare the 27 million-electron-volt energy barrier in the uranium atom!). The tremendous energy of this radiation, as it enters the upper atmosphere and collides with air atoms, results in the formation of a secondary stream of charged particles in great variety.
Before these particles (i.e., the primary cosmic radiation) can reach the earth’s surface they must pass through the atmosphere. The blanket of air covering our planet is heavier than many realize — equivalent to a layer of water thirty-four feet thick. Even the tremendous energy of the primary cosmic rays is not sufficient to enable them to get through this much matter unchanged. However, the debris resulting from their collisions with air atoms does reach the surface of the earth and in fact has been detected several hundred feet underground. This debris, in addition to the protons and neutrons of which the struck atoms are composed, includes mesons, unstable particles associated with nuclear structure that are not very well understood at present, gamma rays, like those given off by radium, only more penetrating, and positive and negative electrons.2
2 Ibid., p. 76.
Although comparatively little of the cosmic radiation actually reaches the earth’s surface at present, that part which does reach it gives intimation of the tremendous energy that certain of its particles contain.
The extraordinary penetrating power of cosmic rays is shown, in the first place, by their ability to pass through the earth’s atmosphere, the absorptive power of which for ionizing radiations is approximately equivalent to one meter thickness of lead. But that is not all. The rays have been detected underground and under water at distances equivalent to 1400 meters of water below the earth’s surface. Only particles with many billions of electron volts of energy could have penetrated to such depths.1
1Samuel Glasstone: Sourcebook on Atomic Energy (2nd Ed., New York, Van Nostrand, 1958), p. 562.
The portion of the cosmic radiation reaching the earth’s surface seems to consist predominantly of highly energetic mesons, along with some neutrons, electrons, protons and photons. Mesons are particles intermediate in mass between electrons and protons, which decay very rapidly into electrons.
The question arises as to what effects might be produced on the earth’s surface if a substantial part of this “hard component” of the cosmic radiation, rather than only a very insignificant part, could reach the earth. It is doubtful whether this question can be answered on the basis of present knowledge, since such an environment is not producible, even in the largest accelerators.2
2 “It is not only astrophysicists who are interested in superenergetic cosmic-ray par-tides. Students of the fundamental constitution of matter would very much like to know what happens when one of these particles strikes an atomic nucleus. . . . Experiments at lower energies such as are available from existing accelerators — indeed, from any accelerator yet envisaged — give no hint as to the behaviour of matter at the fantastically high energies of which we have been speaking.” (Bruno Rossi: “High-Energy Cosmic Rays,” Scientific American, Vol. 201, Nov. 1959, p. 145).
But it does seem highly probable that such an environment, which must have reached the earth’s surface to at least some degree both during the first day of the creation and during the Deluge period and possibly at other times as well, would have had a marked effect on such radioactive elements in particular. The bombardment of these atoms, which are basically unstable anyway, by large amounts of various kinds of particles of extremely high energy could hardly fail to have added to their instability. Or, to put it in another way, the addition of large amounts of external energy into the atomic nucleus would have supplied the needed energy for alpha particles or other groups to overcome the energy barrier normally retaining most of them within the nucleus.
This means that it is not only possible, but highly probable, that the disintegration rates of radioactive elements would have been much higher than at present during at least these two periods of earth history. However, there seems to be no way on the basis of present knowledge by which the magnitude of this increase in rates1 can now be determined.
1 The postulated environment would probably produce a variety of nuclear transmutations in addition to accelerating the disintegration of uranium, thorium, etc. The various elements in each decay chain would also be affected. It is thus not strictly correct to speak of a simple increase in decay rate as resulting from such an environment. However, the net effect is the same . . . namely, an increase in the ratios of “daughter” to "parent” elements in each series.
There may also have been other sources of radiation and energy during these periods. The mere fact that the quantity of actively radioactive material in the earth must originally have been greater than at present would have been one such environmental factor. Also, one of the results of the artificial satellite studies in the higher atmosphere has been to reveal a belt of very high incidence of corpuscular radiation.
This abnormal radiation was found above the level about 450 miles high. The evidence is that:
... the great radiation belt around the earth consists of charged particles, temporarily trapped in the earth’s magnetic field. . . . These studies, in connection with other results of the IGY (the cosmic ray work, in particular), begin to relate a variety of atmospheric and spatial phenomena in an exciting and meaningful way, suggesting that major advances are in process of being made and formulated.2
2 Hugh Odishaw: “International Geophysical Year," Science, Vol. 128, December 26, 1958, p. 1609.
These radiation belts contain far more radiation than that due to the incidence of the cosmic rays.
Above some 1000 km. (this transition altitude being longitude and latitude dependent) the intensity of radiation increased very rapidly with increasing altitude, in a way totally inconsistent with cosmic ray expectations.3
3James A. Van Allen, Carl E. McIlwain, and George H. Ludwig: “Radiation Observations with Satellite 1958E*,” Journal of Geophysical Research, Vol. 64, March 1959, p. 271.
As Dr. J. A. Van Allen, the man chiefly responsible for the discovery of these radiation zones, says:
Up to the points at which the counter jammed, it showed counting rates more than 1,000 times the theoretical expectation for cosmic rays. From the rate of increase and the length of the periods of jamming, we judged that the maximum count probably went to several times this level.1
1James A. Van Allen: “Radiation Belts Around the Earth," Scientific American, Vol. 200, March 1959, p. 44.
The many and diversified electrical and magnetic phenomena in and around the earth’s upper atmosphere are thus extremely interesting, but as yet little understood. Just how they all interact with each other at present, or how they may have acted in the past is not known. It is plain, however, that there is an abundance of rays and charged particles of high energies which, if any substantial portion could reach the earth’s surface, would undoubtedly produce very significant changes in many geophysical processes and phenomena, certainly including those of radioactivity.2
2 A highly radioactive environment such as postulated mav, in addition to accelerating the decay of certain elements, have formed artificial radioactive elements, with various decay rates. The fact that these have not been found in nature may mean either that they just have not yet been found, or else that their initial decay rates were also higher than at present and they have substantially vanished by now. It is only the elements with very long half-lives that have survived the accelerated decay periods.
We conclude, therefore, that a time measurement based on the principle of radioactive decay is in itself quite inconclusive. It is, in the first place, quite reasonable to believe that both parent and daughter elements in each radioactive chain were created at the beginning, probably in “equilibrium” amounts. The amount of originally created radiogenic end-product in each chain is uncertain; it is likely, however, that homologous amounts were created in all such minerals so that all such elements would, when created, give an “appearance” of the same degree of maturity or of age. Furthermore, the intense environmental radiation present in the upper atmosphere could well have resulted in much higher decay rates for the radioactive elements at one or more times in the past.
Thus, by the end of the Creation period, each radioactive mineral would very likely contain a sizeable amount of its radiogenic daughter, though actually but a few days old! Again, at the time of the Deluge, it seems reasonable that the increased radioactivity in the environment would have speeded up all decay processes by some unknown amount. Therefore, even in the relatively rare cases where the radioactive mineral was not disturbed excessively during the intense geologic upheavals of the Creation and Deluge periods, the relative amounts of parent and daughter elements would still be entirely incapable of yielding a valid record of true age, since neither the original amount of radiogenic material nor the changes in past decay rates can now be determined. The only thing reasonably certain is that the present decay rate and present amount of daughter element, if applied in a uniformitarian computation, must result in an age-estimate immensely too great!
AGREEMENT OF AGES FROM DIFFERENT METHODS
It might appear at first that these strictures do not invalidate an estimated age which is based on two or more independent calculations with different materials. Uranium and thorium are often found together in the same mineral, for example, and although calculations of the age are usually discordant, they occasionally agree. With respect even to the case of a mineral containing only uranium, Brown says:
Now there are four different ways we can compute the age of the mineral; namely, from (1) the ratio of lead 206 to uranium 238, (2) the ratio of lead 207 to uranium 235, (3) the ratio of lead 206 to lead 207, and (4) the ratio of helium to uranium. Ideally, all four of these ages should agree, and no estimate can be considered trustworthy unless at least two independent methods (i.e., two of the first three here) agree. But, unfortunately, complicating factors often produce discrepancies in evaluating a given sample.1
1 Harrison Brown: “The Age of the Solar System,*‘ Scientific American, Vol. 196, April 1957, p. 82.
There is even more commonly disagreement between uranium and thorium ages, but again there is occasional agreement.
As more and more evidence was gathered, the lead method began to carry conviction. There could be little doubt when pure thorium minerals associated in the same rocks with pure uranium minerals gave the same absolute age.2
2Ο. B. Muench: “Determining Geologic Age from Radioactivity,’’ Scientific Monthly, Vol. 71, November 1950, p. 300.
There are now known even a few cases where there is agreement between ages obtained by the lead method, the rubidium method, and/or the potassium method.
There is good reason to present the state of progress at this time, since the newer techniques have already provided an indication of their usefulness and simplicity in providing potassium-argon and rubidium-strontium ages that agree for rocks for which the two indicated uranium-lead ages disagree. These measurements have also shown that rubidium-strontium and potassium-argon ages can be made to agree with concordant uranium-lead ages by a suitable choice of half-lives for potassium 40 and rubidium 87. The values so found lie within the large range of values for these two constants, which have been obtained by direct laboratory counting experiments.1
1 Lt. T. Aldrich: “Measurement of Radioactive Ages of Rocks,” Science, Vol. 123, May 18, 1956, p. 871.
Creation of Accordant “Apparent Ages"
But this kind of agreement is exactly what is to be expected on the basis of our deductions as to the past history of the radioactive elements, as originally created and as possibly subject during the Création and Deluge periods to accelerated rates of decay. If any of the radiogenic elements were actually and truly created at the beginning, as seems eminently reasonable, it is most consistent with the perfect, “very good” character of the original Creation to infer that these different radiogenic elements were created in homologous quantities. That is, if two or more such elements were to be included in the same created mineral or group of minerals, their relative amounts would have been the same as their relative rates of origin by radioactive disintegration from their respective “parents.” Furthermore, it is most likely that, if these parents were also created in juxtaposition in the same minerals with them, they and each member of their respective decay chains would have been created and present in their so-called “equilibrium” amounts as now governed by the individual decay rates of the members in the chain.
Skeptics will of course be immediately inclined to discard such a deduction as quite unscientific, in virtue of its being by its very nature unverifiable scientifically. And of course this is true to a degree, since no human experimenter can duplicate or even study processes of creation which are no longer going on. But as a matter of fact the assumption of uniformity is equally unverifiable scientifically as far as past history is concerned. It is only uniformitarian presupposition that decides the assumption of uniformity to be more reasonable than that of original creation!
The writers strongly deny that it is unscientific to postulate a primeval and genuine creation. The two great universal principles of thermodynamics — energy conservation and deterioration — inexorably witness to the scientific necessity of original creation. Nor is it unscientific to accept the Biblical revelation, verified as it has been in countless ways, especially by the testimony of the Lord Jesus Christ Himself, as a true and reliable record of that which man cannot discover without such revelation, namely the events and order of the Creation.
All of this leads to the conclusion that, if it had been possible to make a radioactive time-estimate from these minerals immediately after their creation by the same methods as are now in use, they would have indicated some finite age for the earth, and this age, whatever it may have been, would have been the same for each of the different radiogenic elements in the mineral association. This is the most reasonable conclusion possible on the assumption of a genuine primeval creation as recorded in Genesis.
Concordant Changes in Decay Rates
Consider also the probable effect on the relative rates of radioactivity of the different elements during times when the environment was more radioactive than at present, such as on the first day of the Creation week and during the period of the Deluge. Each element of course has at present a definite value for its “half-life” or rate of disintegration. Whatever may be the fundamental nature and cause of these respective decay processes, it is likely that each would be affected roughly proportionately by any environmental factor potent enough to affect them at all. For example, if the higher incidence of cosmic radiation during any period were such as to have, say, doubled the rate of decay of uranium into lead, it is most probable that it would also have approximately doubled the rate of decay of thorium and that of rubidium and of other radioactive elements. Each rate would have been increased by a factor of the same order of magnitude, since each was subject to the same constant incidence of radiant energy.1
1 Since uranium and thorium have decay chains consisting of several different elements, each with a different half-life, the assumption of simple proportional increase is an over-simplification. The resulting increase of each respective daughter-parent ratio may not be exactly in accordance with our assumption but it should be so qualitatively at least.
And this of course means that, if the particular minerals were left undisturbed, they would continue to yield roughly “accordant" ages, though these ages would now be apparently higher than they appeared at the time of Creation. Similarly, during the Flood period, each decay rate would have been speeded up in the same ratio, so that the individual elements would continue to give “accordant” ages. Finally, at the present date, still assuming this to be one of the relatively rare cases where the minerals have remained comparatively undisturbed through all the vicissitudes of geomorphic history, the suite of minerals would still give accordant ages, but the age so indicated would obviously be much greater than the true age since its creation!
This can all be illustrated by a somewhat simplified1 algebraic calculation which will demonstrate the principles involved. Consider that we have at hand two distinct radiogenic elements, whose rates of production by decay from their parents are denoted by R and cR, with c being the constant ratio of these two production rates to each other. During any specific time interval T, the amounts of the two radiogenic elements produced are therefore R(T) and cR(T). Thus, the total amounts generated in the given time are in the same ratio c as their rates of generation.
1 This discussion is not meant to be an exact exposition of radiogenic age computation; the relation is mathematically more complicated than the direct proportion assumed for the illustration. Nevertheless, the principles described are substantially applicable to the actual relationship.
If these elements existed also as a result of direct creation, it is reasonable to assume that they existed in these same proportions. Say, then, that their initial amounts are represented by quantities of A and cA, respectively. Now, if at some time the incidence of environmental radiation is increased, both rates will be increased in roughly these same proportions; assume that both are multiplied by a factor k and that the increased rates persist throughout a length of time Τ'. Prior to this period, the normal rates applied and persisted, say, for a time T°, and following this period they applied again for a time of T*.
The total quantity of the first element that would now be measured would therefore be: A + R(T°)+k(R)(T')+R(T*). The total quantity of the second element would be: cA + cR(T°)+k(cR) (T')+cR (T*).
Now, if these total quantities of the two elements are each used to make an age-estimate, their respective normal decay rates would of course be used, since it is commonly assumed that these rates could never have been different. Accordingly, the two ages would be calculated as follows:
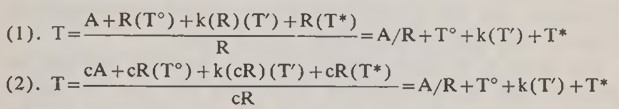
Obviously, these two age-estimates agree perfectly, and might therefore be thought to verify each other and demonstrate the validity of both computations. As a matter of fact, however, each is too large, since the true age of each is only T° + T' + T*. Each is too high by the amount: A/R+-(k — 1) (T'). The numerical value of this excess depends upon the initial amount present A and on the rate increase factor k, and neither of these quantities is known nor evidently can ever be determined. Therefore, it is concluded that it is impossible to make a really certain age determination unless it is known beyond any question that the term A is zero and the factor k is 1, or perhaps some other known values.
Nor does the fact that two or more apparently independent age-estimates agree prove that the computations are valid and the age correct! The foregoing analysis shows that this result is to be expected regardless of whether or not the decay rates had changed in the past, and therefore it proves nothing except that the mineral under examination had probably not been disturbed and its component parts segregated since its original formation.
This apparent agreement is really the only evidence that might be offered to prove that the rates had not varied in the past, as we have already shown. But now we have shown that it does not necessarily prove this at all. Therefore, radioactivity age estimates cannot legitimately be used as proof of the age of the earth or of any formation in it!
Someone may object that it has been proved that the disintegration rate of uranium has never changed during past geologic time, since the size of the so-called “pleochroic halos” is the same in strata of all ages. These halos are spherical zones of discoloration produced in rocks around radioactive nuclei by the ionizing powers of the alpha particles emitted from the nucleus. The distance to which these par-tides can penetrate before they are stopped depends on their energy of emission, and this in turn is believed to control the normal rate of decay, high rates corresponding to large ranges.
The range of the alpha-particles depends, however, not only on the decay rate of the radioactive nucleus but also upon the nature of the material in which it is enclosed, the denser the material, the shorter the range. For this reason, this particular argument is usually limited only to the halos surrounding nuclei of uranium or thorium in a matrix of mica. The argument goes that, for this type of halo, the radius is always the same, and therefore that the disintegration rate must always have been the same.
There is some reason to question this assertion, however. Nearly all the studies that have been made on this subject were made by Joly, about 1907, and G. H. Henderson, in 1934. Others have simply referred to their work and interpreted it as proving the constancy of the decay rate. Joly, however, had himself concluded that the decay rate had changed.
Joly’s study of pleochroic halos in micas of various geological ages brought out a variation of the radii of halos of presumably the same radioactive origin, the older being apparently the longer. His suggestion of varying rate of disintegration of uranium at various geological periods would, if correct, set aside all possibilities of age calculation by radioactivity methods. Fortunately, enough evidence has been found of correct radii for the different geologic periods and sufficient variation in the same period that one is forced to look for a different explanation of such variations as were observed by Joly.1
1 A. F. Kovarik, in The Age of the Earth, Adolph Knopf, Editor, Bulletin 80, National Research Council, 1931, p. 107.
Although this statement explicitly denies that the halos indicate different decay rates, as thought by Joly, it does admit that there is quite a bit of variation in the halo radii, and therefore the claim that they always show the same radii is clearly unwarranted. The most that can be claimed is that they exhibit a rather wide statistical fluctuation about a mean value — which is itself microscopic in size!
More recent studies by a consulting metallurgist, Dr. Roy M. Allen, confirm this variation in radii, together with the difficulty of truly meaningful measurement of them. Among various conclusions regarding the variability in character and occurrence of these halos, the following is of particular interest:
The extent of the halos around the inclusions varies over a wide range, even with the same nuclear material in the same matrix, but all sizes fall into definite groups. My measurements are, in microns, 5, 7, 10, 17, 20, 23, 27, and 33. Joly’s figures correspond with these except he does not include the smaller sizes and does include 39 (38-40) which I have not run across. Halos sometimes show two, or even three definite rings or zones, indicating the presence of more than one radioactive element, each with its own specific alpha-ray path.1
1 Roy M. Allen: “The Evaluation of Radioactive Evidence on the Age of the Earth,” Journal of the American Scientific Affiliation (December 1952) p. 18.
In view of these observations made by a very careful scientist, it appears that the oft-reiterated claim about the constancy of the radius may be invalid. Therefore, there remains no actual evidence that the decay rates may not have been different at some time or times in the past than they are at present.
But even if there should turn out to be at least a statistical constancy of the halo radii, this does not mean that the past rates are the same as present rates. According to our hypothesis, all rocks are of essentially the same age, so that the fact that pleochroic halos have about the same radius in all of them is exactly what would be expected. They were all formed at about the same time; therefore, the same decay rates, whether constant or changing, have continually occurred in all of them. Furthermore, it seems unlikely that even a substantial increase in the decay rate would cause any measurable change in the halo radius. The latter is determined mainly by the extremely short-lived elements in the decay chain, for these have the longer ranges. It does not seem necessary to conclude that an acceleration of the first stage in the decay process — the expulsion of helium atoms from the uranium nucleus — would thereby accelerate all other stages in the chain individually. But even if it did, the increase in alpha particle range corresponding to increase in expulsion energies becomes vanishingly small as the energies increase, and this factor would prevent any very substantial increase in the radius.
This argument, however, is not infallible, because according to the bilogarithmic form of the law of Geiger and Nuttall a considerable variation in the decay constant will produce a very small change in the range of the alpha-particle.1
1 Kalervo Rankama: Isotope Geology (New York, McGraw-Hill, 1954), p. 109.
Thus, we conclude that a statistical constancy of the halo radii in rocks of various “ages” proves nothing about the decay rates.
SUPPOSED CORRELATION OF RADIOACTIVITY AND STRATIGRAPHIC AGES
There is still the claim to be faced that the radioactivity age-estimates agree in general with the geological ages assigned to the strata on the basis of paleontology and stratigraphy. That is, the “absolute ages” deduced from radioactivity measurements for various positions in the geologic time scale fall into proper position, so that strata deemed young on the basis of paleontology give young radioactivity ages, paleontologically old strata yield higher ages, etc. On this basis, a scale of absolute time has been worked up for the entire geologic column and, in various forms, has been published in many, many books and periodicals. For example, Arthur Holmes, probably the most prolific of all writers and workers in this field, said long ago in the famous National Research Council symposium on geochronology:
In attempting to build up a time scale it is clear that we have to steer a difficult course through a maze of data of very variable quality, guided in some places by atomic weight evidence, in others by series of accordant ratios, but in far too many by a subjective weighing of probabilities. Nevertheless, although only a few points can be fixed with precision into the geological column, and the total assemblage of data is too confused to permit detailed accuracy, it is remarkable how consistently the most probable ratio for each of the various suites falls into its proper place and order as judged by geological age.2
2 Arthur Holmes, in The Age oi the Earth, Adolph Knopf, Editor, Bulletin 80, National Research Council, 1931, p. 431.
A major reason for the supposed concordance between the radioactivity and paleontological time scales is evident from this remarkable quotation: the time estimates which agree with the pre-judged proper order are accepted, the others are rejected! The latter are supposed to have been altered in some way since deposition and therefore unacceptable, the criterion for postulating alteration being this lack of agreement. This sort of “subjective weighing of probabilities” is quite convenient, but hardly constitutes compelling proof.
But it will be objected that the above was written almost thirty years ago; great masses of data have been accumulated since then from radioactive minerals from all parts of the world and all parts of the geologic column. Listen, then, to the recent words of Adolph Knopf (who was also editor of the symposium cited above) in a recent review of the data:
An urgent task for geology is to determine, in years, the length of the eras, periods, and “ages” (time spans of the stages) and, eventually of the zones. Not a single one of them — eras, periods, and ages, let alone zones — has yet been reliably determined. This statement is possibly surprising in view of the fact that almost any modern writer can produce a geologic timetable that gives precise datings and lengths of the eras and systems and even of some of the smaller subdivisions. . . . These figures have been obtained in various remarkable ways. Ultimately, however, they are tied to three dates based on atomic disintegration: 60 million years, the age of the pitchblende at Central City, Colorado; 220 million years, the age of the pitchblende at St. Joachimstal, Bohemia; and 440 million years, the age of the uranium-bearing shale at Gullhogen, Sweden. The age of the Swedish shale is the only one of these that is paleontologically controlled. . . . All other absolute ages have been derived from the three radioactive tie points by interpolation based on thicknesses of strata or by “reasoned guesses” . . .1
1 Adolph Knopf: “Measuring Geologic Time,” Scientific Monthly, Vol. 85, November 1957, p. 227.
Now, herein is a marvelous thing! Consider what science has proved! All the world of learning and scholarship has been driven to accept the fact of universal evolution as the basic principle and philosophy controlling everything, despite the testimony of both Scripture and the demonstrated truths of energy conservation and degradation, because of the supposed overwhelming weight of scientific evidence. When one goes to the geneticist to see such evidence, he is shown only micromutations and is directed to the geologist for evidence of historical evolution on the broader scale. The geologist then points to a series of time-rock units, which has been erected on the assumption of organic evolution, despite the evidence of many exceptions and contradictions in the series, and which even at best still contains essentially the same gaps that the genetic evidence shows. Although most of these rocks show evidence of rapid, catastrophic formation, he maintains that radioactivity has provided him with a scale of absolute time that proves that they are in the proper order and that the times are so immense as to provide for all the statistical improbabilities that evolution demands. And when we inquire into the nature of the radioactive evidence that proves such wonderful things, we learn that out of the hundreds and hundreds of such measurements that have been made on rocks from every geological age and from all parts of the world, after winnowing out all those with discordant ratios, with anomalous amounts of component elements, or that disagree with the paleontological dating, there are three (three!) that form the basis of the time-scale and that all others are interpolated therefrom by “reasoned guesses,” based mainly on relative thicknesses of strata.
And of these three datings, only one is considered adequately dated paleontologically. That one, the Cambrian shales of Sweden containing nodules of uranium called “kolm,” has long been the pride and joy of geochronologists. But it also is highly questionable. Knopf says:
The isotopic composition of the radiogenic lead in the kolm was determined by Nier, in 1939, and yielded the very disconcerting result that the age, based on Lead 206-Uranium 238, is 380 million years, whereas that based on Lead 207-Lead 206 is 770 million years. Now Nier, it must be recalled, regarded the figure given by the Lead 207-Lead 206 ratio as being the least subject to error and hence the most reliable. For the kolm, however, the figure 770 million years was clearly too large.1
1 Knopf, op. oil., p. 234.
However, instead of rejecting this as discordant, the discrepancies have been compromised and the age recorded as 440 million years, on the assumption that some of the radon gas formed as one stage in the decay series had escaped, thus causing too small an amount of radiogenic lead to be produced.2 Note that there is no proof that this was actually the case; it merely was an assumption which provided a means of reconciling the discrepancy and arriving at an age that seemed appropriate for the paleontological stratum in which the mineral was found.
2 Ibid. More recent measurements on this material, by J. C. Cobb and J. L. Kulp, indicate that "preliminary measurements of radon leakage show room-temperature radon loss to be of an order of magnitude less than that needed to explain the discordance” ("Age of the Swedish Kolm," Bulletin of the Geological Society of America, Vol. 68, Dec. 1957, p. 1711).
And this date, deduced by so devious and questionable an analysis, is considered the best and most reliable of all the hundreds and perhaps thousands of dates that have been obtained from the radioactivity measurements on the earth’s post-Precambrian strata!
Still more recently, Henry Faul concludes that only the Colorado pitchblende is at all acceptable:
Of the five points on which Holmes based his time scale, only one (Laramide) can be included now. The stratigraphically unimpeachable “Swedish Kolm” from the alum shale does not present a closed system, and all attempts to establish an age for it have failed. The stratigraphic limits on Holmes’ remaining three points are too vague to make them useful.1
1Henry Faul: “Geologic Time Scale," Bulletin, Geological Society of America, Vol. 71, May 1960, p. 640.
With regard to the device of interpolating dates for other geologic horizons from thicknesses of strata, Knopf says:
As long ago as 1936 the conclusion had been reached by Twenhofel [the outstanding authority on sedimentation] that estimates of time based on thicknesses of strata “are hardly worth the paper they are written on,” and he presents detailed evidence in support of this revolutionary concept.2
2 Knopf, op. cit., p. 228.
Thus, the general inadequacy of the radioactivity geochronometric data for paléontologie dating is indicated by Teichert:
The literature contains few age determinations (perhaps no more than one) on syngenetic radionuclides from paleontologically defined stratigraphic units, and almost all radioactive age determinations are made on igneous, hydrothermally introduced, or secondarily transported minerals that cannot as a rule be referred to a precisely defined place in the stratigraphic succession. At present, no coherent picture of the history of the earth could be built on the basis of radioactive datings.3
3 Curt Teichert: “Some Biostratigraphical Concepts,” Bulletin of the Geological Society of America, Vol. 69, January 1958, p. 102. Henry Faul says: “When we now attempt to construct a time scale by reasonable interpolation between these points, it becomes obvious that the available data are still too few, too poor, and internally inconsistent” (Op. cit., p. 642).
On the other hand, we do recognize, of course, that in spite of the high degree of confusion and inconsistency in much of the radio-activity age data, there does seem to be a certain rough tendency for some degree of correlation between paleontologic and radioactivity relative ages. The bulk of these measurements have been made on Pre-Cambrian strata, of course, and although there are many flagrant exceptions, most of the values so obtained do indicate ages greater than 500,000,000 years, which is now assumed to be about the beginning of the Paleozoic Era.1 Similarly, a number of age estimates obtained on the fossiliferous strata, especially as obtained within the last few years by the Potassium-Argon method, exhibit rough trends parallelling the traditional order of the geologic column.
1 However. Henry Faut says: “K Ar and Rb Sr determinations on intrusive rocks of the Paleozoic era almost always give ages greater than the numerical ages predieted by the currently accepted time scale. . . . The results show that one may begin to think of fairly drastic revision in the Paleozoic time scale.” (“Doubts of the Paleozoic lime Scale,” American Geophysical Union Program Abstracts, May 1959, p. 42.)
Thus, although we insist that the case in favor of the accepted geologic time-scale has been made to appear much stronger than it is by this dubious process of accepting those data which support it and rejecting those which contradict it, there still seems to remain enough evidence of correlation to indicate some basic physical phenomenon which has operated in such fashion as to cause apparently higher proportions of radiogenic materials in the “older” strata, that is, those which were usually deposited earlier and deeper than the others.
Cause of the Apparent Limited Agreement
But again, isn’t this tendency only what is to be expected on the basis of the events inferred from Scripture to have transpired during the periods of the Creation and the Flood? At the time of the primal Creation, each of the radioactive parent elements was created in place at various points throughout the crust. As we have already indicated, it is reasonable that there would also be associated with each parent atom an “equilibrium amount” of its various daughters. But we must allow for the probability that there were intense crustal disturbances and adjustments during the first days of the Creation period. It is probable also that certain amounts of non-radiogenic lead, helium, argon and other of the elements associated with the disintegration chains were created initially, independently of the equilibrium amounts established in association with radioactive parents. During the later Creation stages, as well as during the Deluge, there would be abundant opportunity for mixing of the “common” isotopes and their sister “created radiogenic” isotopes, as well as with the “actually radiogenic” isotopes which began to form immediately after the creation of the parents.
Some such mixing process as this is envisaged by Faul, who says:
It is very likely that “primordial lead,” or the lead that was made with all the other elements at the time of nucleogenesis, was well mixed. When the earth’s crust was formed, the primordial lead was frozen into rocks that also contained uranium and thorium in various ratios to lead.1
1 Henry Faul: Nuclear Geology (New York, John Wiley & Sons, 1954), p. 297.
Therefore, it would be expected that those radioactive minerals found in the rocks of the shields and other Pre-Cambrian formations would yield many different age values, though in general most of them would be very high. This is exactly what is found.
With regard to the sedimentary strata, as well as the igneous intrusions found in them, together with the other fossiliferous volcanic rocks, these we believe were largely formed during the Deluge, as outlined in the preceding chapter. The materials for these rocks were derived from the primitive crustal rocks in large part, although there must undoubtedly have been a primitive soil created as well to support the first life-forms, and these materials also were eroded and redistributed by the flood waters. Mixing of radiogenic and non-radiogenic isotopes must have been even more intensive during the Flood period than during the Creation period.
As a general rule, those radioactive minerals nearest the surface would be subject to the greatest degree of mixing during the Flood, since they would have been those first eroded by the torrential rains and swollen streams. This would have had the effect of “diluting” the radiogenic component of such minerals, making those near the surface appear to be relatively “younger” than those further below the surface. Furthermore, both during and after the Deluge, those minerals nearer the surface and in the lighter, less consolidated sediments, would be much more likely to lose their gaseous components (e.g., argon from the potassium minerals, radon and helium from the uranium) than those in the denser, deeper rocks. This, too, would have the effect of making the radioactive minerals in the surface rocks appear to be younger than those below. Obviously, with all the intense mixing involved, the inferred orders would represent only rough trends rather than inviolable rules, and this is exactly the state of things encountered in the present strata.
Also, there are many radioactive minerals found in the igneous intrusions in the sedimentary strata, which we have inferred to be associated with outpourings from the “fountains of the great deep" during the Flood. These radioactive minerals would also, in general, contain smaller relative amounts of radiogenic elements because of the greater mixing and diffusive action associated with the intrusion and would therefore, when deposited, read “younger” ages than those in the true Pre-Cambrian strata.
Further discussion of other aspects of the radioactivity age estimates does not appear necessary here. The important features of these data are all now seen to be explainable in terms of the phenomena and activity associated with the Creation and the Deluge. It is not at all necessary to interpret them as teaching the immense ages hitherto inferred therefrom. In fact the gross and entirely unwarranted assumptions on which they are based (especially uniformity and denial of any true creation), in contrast to the sound basis in Holy Scripture upon which the assumptions in our interpretation are based, justify the assertion that the latter is actually much better oriented scientifically than the former.
ASTRONOMIC METHODS OF AGE MEASUREMENT
Nor do we need to consider at length the various other methods that have been used for estimating the age of the earth and the universe. We can say in general that they are based on much more extreme assumptions and on much flimsier empirical evidence than are the radioactivity methods. For example, a commonly heard claim is that the rate of expansion of the astronomic universe is such as to indicate a time since the beginning of the expansion of about five billion years, a time which is thought to be compatible with radioactivity evidence as to the age of the earth's crust. But, as the astronomer, Dr. T. S. Jacobsen of the University of Washington says:
... the current estimates for the expanding universe, whether on the old or the new time scale, are very far from being in any sense factual. While it is true that the Hubble constant enters into the computation of the “age,” McVittie has stressed that a factor depending upon the model, a pure guess that the present radius of curvature is about 100 times the original Einstein radius, and an assumption of the average density of matter in the observed universe (an estimate which is still uncertain within a factor of 1000 according to some observational astronomers) all enter into the computation of the age. In addition to these uncertainties, we do not know that the nebulae have always moved at their present constant speeds. Accelerations and decelerations with time are at present being considered as possibilities. The result is that we know nothing certain about the age of the universe.1
1 T. S. Jacobsen: Review of “Space, Time, and Creation,” by Μ. K. Munitz, appearing in Science, Vol. 128, September 5, 1958, p. 527.
A common opinion is that the very distance of the far galaxies testifies that the universe must be billions of years old. Since these galaxies are known to be some few billion light-years away, by definition it has taken that number of years for their light to reach us; therefore they are at least that old, so the argument goes.
But this contention of course again begs the question. It constitutes an implicit denial that the universe could have been created as a functioning entity. If creation has occurred at all (and the two principles of thermodynamics require this) then it is reasonable that it would have been a complete creation. It must have had an “appearance of age” at the moment of creation. The photons of light energy were created at the same instant as the stars from which they were apparently derived, so that an observer on the earth would have been able to see the most distant stars within his vision at that instant of creation. There is nothing unreasonable either philosophically or scientifically in this, although it does contradict the uniformitarian assumption.
Even apart from this factor, it is not commonly realized how many esoteric assumptions enter into even such apparently simple concepts as the speed of light and the geometric nature of the universe. To illustrate, a recent theory rather vigorously advocated by some astrophysicists strongly questions the constancy of the velocity of light in space and time, as well as the generally accepted Einsteinian nature of the universe. These writers regard the universe much more realistically as a Euclidian universe (3-dimensional, as in our everyday experience) and the velocity of light as constant with respect to its source, rather than with respect to any observer as Einstein does. Among the implications of this thesis the following is most interesting:
In essence, therefore, the method of this paper leaves astronomical space unchanged but reduces the time required for light to travel from a star to the earth.1
1Parry Moon and Domina Eberle Spencer: “Binary Stars and the Velocity of Light,’’ Journal of the Optical Society of America, Vol. 43, August 1953, p. 639.
Or, more specifically, and rather surprisingly:
The acceptance of Riemannian space allows us to reject Einstein’s relativity and to keep all the ordinary ideas of time and all the ideas of Euclidean space out to a distance of a few light years. Astronomical space remains Euclidean for material bodies, but light is considered to travel in Riemannian space. In this way the time required for light to reach us from the most distant stars is only 15 years.2
2 Ibid., p. 635.
We do not propose to evaluate this theory but only to point out that all cosmological theory is still highly speculative. The very fact that such a theory can be developed and seriously considered demonstrates that astronomy has nothing really definite as yet to say about the age of the universe. And this is entirely aside from the really much more fundamental issue of the reality of a genuine Creation!
There are many other geochronometers that have been suggested and utilized to a certain extent, but each is based on the typical uniformitarian assumptions, and none have been as widely and intensively developed as have those already discussed. Each of them has grave deficiencies and is admittedly less reliable than the radioactivity methods which we have already analyzed and reinterpreted.
THE RADIOCARBON DATING OF RECENT DEPOSITS
We must give some consideration, however, to one more particular method, namely the radiocarbon method of dating. This tool has become quite widely used and accepted in recent years and is important to our study since it professes to supply absolute dates for events within the past 30 or 40 thousand years. This of course covers the apparent periods of Biblical history, as well as more recent dates, and so bears directly upon the question of the Flood and other related events.
The method was first developed by W. F. Libby in 1946. Since that time, literally thousands of such measurements have been made, by workers in many different laboratories, and a great variety of archaeological and Recent geological datings have been obtained. The formation of radiocarbon (that is, Carbon 14, the radioactive isotope of ordinary carbon) from cosmic radiation was first discovered, however, by Serge Korff, an authority on cosmic rays. Describing the Carbon 14 dating method which has resulted, Korff says:
Cosmic ray neutrons, produced as secondary particles in the atmosphere by the original radiation, are captured by nitrogen nuclei to form the radioactive isotope of carbon, the isotope of mass 14. This isotope has a long half-life, something over 5500 years. By the application of some very well thought-out techniques, Libby and his colleagues have actually not only identified the radiocarbon in nature, but have also made quantitative estimates thereof. Since this carbon in the atmosphere mostly becomes attached to oxygen to form carbon dioxide, and since the carbon dioxide is ingested by plants and animals and is incorporated in their biological structures, and further, since this process stops at the time of the death of the specimen, the percentage of radiocarbon among the normal carbon atoms in its system can be used to establish the date at which the specimen stops metabolizing.1
1Serge A. Korff: “The Origin and Implications of the Cosmic Radiation," American Scientist, Vol. 45, September 1957, p. 298.
There is no doubt that this constitutes a very ingenious and powerful dating tool, provided only that the inherent assumptions are valid. Kulp lists the assumptions as follows:
There are two basic assumptions in the carbon 14 method. One is that the carbon 14 concentration in the carbon dioxide cycle is constant. The other is that the cosmic ray flux has been essentially constant — at least on a scale of centuries.2
2J. L. Kulp: “The Carbon 14 Method of Age Determination," Scientific Monthly, Vol. 75, November 1952, p. 261.
To which we might add the assumption of the constancy of the rate of decay of the carbon 14 atoms, the assumption that dead organic matter is not later altered with respect to its carbon content by any biologic or other activity, the assumption that the carbon dioxide content of the ocean and atmosphere has been constant with time, the assumption that the huge reservoir of oceanic carbon has not changed in size during the period of applicability of the method, and the assumption that the rate of formation and the rate of decay of radiocarbon atoms have been in equilibrium throughout the period of applicability. Every one of these assumptions is highly questionable in the context of the events of Creation and the Deluge.
But it is maintained that the method has been verified beyond any question by numerous correlations with known dates. Here an observation by Libby himself is interesting and in point:
The first shock Dr. Arnold and I had was that our advisors informed us that history extended back only 5000 years. We had thought initially that we would be able to get samples all along the curve back to 30,000 years, put the points in, and then our work would be finished. You read books and find statements that such and such a society or archaeological site is 20,000 years old. We learned rather abruptly that these numbers, these ancient ages, are not known; in fact, it is at about the time of the first dynasty in Egypt that the last historical date of any real certainty has been established.1
1W. F. Libby: “Radiocarbon Dating," American Scientist, Vol. 44, January 1956, p. 107.
It is obvious, therefore, that any genuine correlation of the radiocarbon method with definite historical chronologies is limited only to some time after the Flood and Dispersion. The major assumptions in the method are evidently valid for this period, but this does not prove their validity for more ancient times, the periods in which we would infer that the assumptions are very likely wrong and therefore the datings also wrong.
Attempts to apply the carbon 14 method to earlier datings have, in fact, been called in serious question by geologists for entirely different reasons than our own. Charles B. Hunt, who is recent president of the American Geological Institute, has cautioned:
In order that a technique or discipline may be useful in scientific work, its limits must be known and understood, but the limits of usefulness of the radiocarbon age determinations are not yet known or understood. No one seriously proposes that all the determined dates are without error, but we do not know how many of them are in error — 25%? 50%? 75%? And we do not know which dates arc in error, or by what amounts, or why.2
2Charles B. Hunt: “Radiocarbon Dating in the Light of Stratigraphy and Weath-cring Processes," Scientific Monthly, Vol. 81, November 1955, p. 240.
Hunt emphasizes particularly the danger of contamination of the sample by external sources of carbon, especially in damp locations.
The sharp reduction in previously estimated dates for the close of the glacial period (a date which had been estimated mainly on the basis of counts of varved clays presumably laid down by the retreating ice sheet) has been a source of much argument among Pleistocene geologists as to the relative merits of the varve method (which gave a date of over 20,000 years) and the radiocarbon method (which gave a date of about 11,000 years). The American specialist in varve chronologies, Dr. Ernst Antevs, has sharply criticized the radiocarbon method, as a result:
In appraising C 14 dates, it is essential always to discriminate between the C 14 age and the actual age of the sample. The laboratory analysis determines only the amount of radioactive carbon present. . . . However, the laboratory analysis does not determine whether the radioactive carbon is all original or is in part secondary, intrusive, or whether the amount has been altered in still other irregular ways besides by natural decay.1
1 Ernst Antevs: “Geological Tests of the Varve and Radiocarbon Chronologies,” Journal of Geology, March 1957, p. 129.
A conference on radiocarbon dating held in October, 1956, resuited in the following conclusions about the reliability of the method:
Local variation, especially in shells, can be highly significant. Possible variations in the size of the exchange reservoir under glacial climates are unimportant. The most significant problem is that of biological alteration of materials in the soil. This effect grows more serious with greater age. To produce an error of 50 per cent in the age of a 10,000 year old specimen would require the replacement of more than 25 per cent of the carbon atoms. For a 40,000-year-old sample, the figure is only 5 per cent, while an error of 5000 years can be produced by about 1 per cent of modem materials. Much more must be done on chemical purification of samples.2 The problem of atmospheric contamination by fossil fuels has also come in for some consideration, since the burning of coal and oil during the past century and more has added measurably to the amount of carbon dioxide in the carbon cycle. A recent study on the quantitative aspect of this factor concludes:
2 F. Johnson, J. R. Arnold, and R. F. Flint: “Radiocarbon Dating,” Science, Vol. 125, February 8, 1957, p. 240.
... it follows that atmospheric carbon dioxide has probably been diluted to the extent of about 314 percent with carbon dioxide from the combustion of fossil fuels. The radiocarbon evidence indicates, on the basis of a comparison of the radiocarbon assays of old, historically dated marine shells from the Atlantic coast with the assays of their modern counterparts, that there has been a perceptible dilution of shallow oceanic carbonates with dead carbon from fossil fuels. The limited data available suggest that the extent of dilution is possibly one to two per cent.1
1 H. R. Brannon, A. C. Daughtry, D. Perry, W. W. Whitaker, and M. Williams: “Radiocarbon Evidence on the Dilution of Atmospheric & Oceanic Carbon,” Transactions, American Geophysical Union, Vol. 38, October, 1957, p. 650.
This means that the standard figures as to present content of carbon dioxide in the exchange reservoir of carbon, on which radiocarbon age calculations are based, are incorrect with respect to conditions under which older specimens were formed and have since been decaying. Although this might be corrected approximately by modifying the standard to one before the Industrial Revolution, the following caution is also in order:
Since completion of the present list, a careful study has been made of a series of samples of known age. It was found that the activity of radiocarbon in the atmosphere was going up and down even before the Industrial Revolution.2
2 H. deVries and H. T. Waterbolk: “Groningen Radiocarbon Dates HI,” Science, Vol. 128, December 19, 1958, p. 1551.
This particular correction, however, is only of the order of a few hundred years for most computed dates, so apparently is negligible for the purposes of our studies. Much more important are the effects of the aforementioned assumptions in the method,3 when viewed in the light of the probable events occurring during and immediately after the Flood.
3 Another important source of error is the assumed contemporary assay, the initial concentration of radiocarbon in the material, which can be seriously diluted by old carbon in the environment at the time the organism was living, thus making the computed radiocarbon age too high. “Any error in the choice of the value of the contemporary assay results in an error of the radiocarbon age. . . . The error in age is approximately 80 years for an error in contemporary assay of one percent and is proportionately more for larger errors in contemporary assay.” (W. W. Whitaker, S. Valastro, Jr., and Milton Williams,' “The Climatic Factor in the Radiocarbon Content of Woods,” Journal of Geophysical Research, Vol. 64, August 1959, p. 1023).
CARBON 14 AND THE DELUGE
Antediluvian Radiocarbon Proportions
Prior to the Flood, it is highly probable that the ratio of ordinary carbon to radiocarbon in the atmosphere was much higher than at present, mainly because of the global semi-tropical climate and the vast amounts of plant life found around the world. This effect would have been augmented by the smaller amount of carbon sustained in the ocean then than now, since the oceans were smaller and the land areas larger before the Flood. And it is possible that it would be still further augmented by the shielding effect of the thermal vapor canopy, which would have inhibited the formation of radiocarbon in the high atmosphere. All of these factors would have reduced the ratio of radiocarbon to ordinary carbon to a much smaller fraction than now obtains.
Another possible effect of the vapor canopy is very interesting. In addition to the formation of Carbon 14 from nitrogen in the atmosphere by cosmic-ray neutrons, these neutrons also react with deuterium (heavy hydrogen, the hydrogen isotope in heavy water), which would undoubtedly have been present in substantial amounts in such a canopy, to form tritium, a still heavier isotope of hydrogen. Tritium is unstable and decays rapidly by beta decay to an isotope of helium, He 3. But it turns out that there is too much He 3 in the atmosphere to be accounted for by this process operating at present rates during geologic time. The cosmic ray authority, Korff, suggests the following solution of the problem:
There are two factors which would tend to increase the amount of tritium. One of these is that the intensity of cosmic radiation, and hence the rate of production of neutrons might have been higher at some time in the geologic past. . . . The second possibility invoking action in the past assumes that at a time when the earth was warmer the atmosphere contained much more water vapor, and (the process of generating tritium from deuterium) might have been operating at a much higher rate than at present.1
1Serge A. Korff: “Effects of the Cosmic Radiation on Terrestial Isotope Distribution,” Transactions, American Geophysical Union, Vol. 35, February 1954, p. 105.
The vapor canopy thus not only provides an explanation for the present excess of atmospheric Helium 3 but also implies that the proportion of cosmic ray neutrons reacting with nitrogen to form radiocarbon would be smaller by the amount reacting thus with the hydrogen. This factor combines with the others mentioned to assure that the per cent of radiocarbon in the carbon dioxide of the antediluvian atmosphere must have been much smaller than at present. Therefore the radioactivity of living organisms ingesting this carbon dioxide would have been much smaller than that of organisms living at present.
Thus, antediluvian organic matter probably would now have little or no radioactivity if preserved as fossils, even though they may actually have been buried by the Flood only a few thousand years ago. Although present radiocarbon activity seems to indicate measurements will detect radioactivities from matter perhaps as much as 70,000 years old, such indications are based upon the assumption of uniformity. This stricture is considered quite serious by Dr. G. N. Plass, who is a specialist in investigations dealing with atmospheric carbon dioxide:
All calculations of radiocarbon dates have been made on the assumption that the amount of atmospheric carbon dioxide has remained constant. If the theory presented here of carbon dioxide variations in the atmosphere is correct, then the reduced carbon dioxide amount at the time of the last glaciation means that all radiocarbon dates for events before the recession of the glaciers are in question.1
1Gilbert N. Plass: “Carbon Dioxide and the Climate." American Scientist, Vol. 44, Inly 19x6. p. 314.
Postdiluvian Radiocarbon Proportions
With respect to plants and animals living after the Flood, the loss of the earth’s canopy would tend to increase the per cent concentration of Carbon 14 in the carbon dioxide of the atmosphere, since the rate of formation of Carbon 14 atoms would be accelerated by the loss of the canopy. On the other hand, the influx of carbon into the atmosphere from the intense volcanism during and after the Deluge period must have greatly augmented the carbon dioxide content of the atmosphere and oceans as well, probably more than offsetting the increase in C-14, at least for some time.
Furthermore, the equilibrium condition between generation and decay of radiocarbon, which has to be assumed in making any age calculation by this method, would obviously not be applicable for quite a long time after the Deluge. Although there quite probably was a marked increase in rate of formation of Carbon 14 atoms at the time of the Deluge due to the greater effectiveness of the cosmic radiation in this process after the precipitation of the vapor canopy, it would necessarily have taken many years for the total amount of radiocarbon to have built up a reservoir of such size that the numbers of atoms being created and dissipated were equal. And this would mean that organisms living in these early years and centuries after the Flood would have received a proportionately smaller amount of radiocarbon into their systems than those living in later times. Especially in the few hundred years immediately after the Flood, during the time when mixing of the atmospheric, oceanic, and biologic carbon was first being accomplished, would this be true. In his definitive book on the subject, Libby says:
If one were to imagine that the cosmic radiation had been turned off until a short while ago, the enormous amount of radiocarbon necessary to the equilibrium state would not have been manufactured and the specific radioactivity of living matter would be much less than the rate of production calculated from neutron intensity.1
1 W. F. Libby: Radiocarbon Dating (Chicago, University of Chicago Press, 1955). P. ?.
The obvious conclusion is that plants and animals living in the early centuries after the Flood would have much less radioactivity than would be assumed on the basis of present rates and therefore would appear to be older than they are.
The specific radioactivity increased as time went on, approaching the present equilibrium rates. That is why radiocarbon dates for the last four thousand years seem to show a generally good correlation with historically verified chronology, although there are many discrepancies and a large margin of error the farther back in time comparisons are made. But for earlier dates, the specific radioactivity in the terrestrial environment becomes progressively smaller as one goes back in time. Therefore, when material older than, say, about four thousand years is analyzed now for radiocarbon, it would certainly be found that the activity was low and, if the age were then calculated on the basis of present equilibrium conditions and rates, it would necessarily be measured to be too old, with the amount of error increasing progressively with the age of the material.
Therefore, the Deluge and associated events adequately explain the data from Carbon 14 studies, accounting for the agreement with historically dated recent events but at the same time indicating that the earlier unverified datings must be too high, as one would infer from the Biblical records.
Consequently, all of the more important of the data from radioactivity methods of geochronometry harmonize perfectly with the Biblical records and inferences associated with the Creation and the Flood. Space does not warrant discussion of all the methods that have been used or suggested, but only those which have been considered the most important and best established. It would be possible by similar analyses to show the essential harmony of the data from these other subsidiary methods (e.g., the ionium method, the varve chronologies, thermoluminescence, etc.) with the Biblically established facts of a genuine recent Creation and universal Deluge.
These events must be dated only some few thousands of years ago according to the Bible, and the evidence that has been brought against this testimony has now been shown rather to harmonize quite satisfactorily with it. In fact, it would seem highly probable that no method of geochronometry could be devised that would permit determination of dates earlier than the Flood, since all such processes, whether geological or meteorological, would almost certainly have been profoundly disturbed and altered by the events of that global cataclysm. The Scriptural description is that “the world that then was, being overflowed with water perished” (II Peter 3:6), and the context shows that this statement comprises the geological earth and the atmospheric heavens! The only possible way in which men can know the age of the earth is by means of divine revelation!
CONTRADICTIONS IN GEOCHRONOLOGY
Even aside from the Biblical testimony against the radioactivity age estimates for the earth and its formations, there are numerous evidences in geology itself against the validity of these tremendous time durations. The currently accepted figure for the age of the earth as deduced from radioactivity of uranium and other elements is about five to six billion years,1 with the solidification of the crust dated about 41/2 billion years ago.
1G. P. Kuiper: “Origin, Age, and Possible Ultimate Fate of the Earth,” in The Earth and Its Atmosphere, D. R. Bates, Ed. (New York, Basic Books, Inc., 1957) p. 14-16.
But there are many geological processes which appear to be at least as suitable for geochronometric purposes as the phenomena of radioactivity and which give much lower estimates than this. None of these is sufficiently precise for accurate measurements, and all involve the same sort of unlikely assumptions as the radioactivity methods, but they are nevertheless sufficiently meaningful to cast very serious doubt on the reliability of the radioactivity estimates.
One of these lines of evidence is derived from the study of meteorites and comets, of which there are large numbers in our solar system. A tremendous amount of meteoritic material falls each year on the earth. Estimates vary widely, but the most careful studies have been made by Hans Pettersson of the Swedish Oceanographic Institute.
Pettersson calculated that the total quantity of dust of meteor origin in the atmosphere, up to a height Of 60 miles, amounts to 28,600,000 tons. . . . half the total — 14,300,000 tons of such dust — settles to earth each year and 14,300,000 tons of new dust must enter the atmosphere.1
1 Isaac Asimov: “14 Million Tons of Dust Per Year,” Science Digest, Vol. 45, Jan. 1959, p. 34. Pettersson confirms this: “If meteoritic dust descended at the same rate as the dust created by the explosion of the Indonesian volcano Krakatoa in 1883, then my data indicate that the amount of meteoritic dust landing on the earth every year is 14 million tons.” (“Cosmic Spherules and Meteoritic Dust,” Scientific American, Vol. 202, February 1960, p. 132).
The significance of this large amount of meteoritic dust, in terms of the supposed great age of the earth, is noted by Asimov as follows:
Of course, this goes on year after year, and the earth has been in existence as a solid body for a good long time, for perhaps as long as 5 billion years. If, through all that time, meteor dust had settled to the earth at the same rate it does today, then by now, if it were undisturbed, it would form a layer 54 feet thick over all the surface of the earth.2
2Ibid., p. 35.
Obviously, no layer of meteoritic dust of any appreciable thickness, certainly not 54 feet, is found around the earth’s surface, although some indications of such a layer have been found on the ocean bottoms.
Pettersson and Rotschi have found good evidence from the peculiar nickel content of deep sea deposits both in the Atlantic and the Pacific Ocean that several thousand tons per day of meteoritic material are accumulated by the earth.3
3 F. L. Whipple, in Advances in Geophysics (Academic Press, Inc., 1952), p. 131.
The absence of this meteoritic dust layer on the earth’s surface cannot be reasonably accounted for in terms of crustal mixing processes, as Asimov claims. This type of material is composed mostly of iron, with large amounts of nickel and other relatively rare components of the earth’s crust, and these elements are not found in sufficient abundance to correspond to the amount supposedly accreted by meteoritic showers. For example, the average nickel content of meteorites is of the order of 2.5 percent, whereas nickel constitutes only about 0.008 percent of the rocks of the earth’s crust.1 Thus, about 312 times as much nickel per unit volume occurs in meteorites as in the earth’s crust. This means that the 54 ft. thickness of meteoritic dust would have to have been dispersed through a crustal thickness of at least 312 x 54 ft., or more than three miles, to yield the present crustal nickel component percentage, even under the impossible assumption that there was no nickel in the crust to begin with! Similar calculations could be made for cobalt and other important constituents of meteorites, all testifying that there simply cannot have been meteoritic dust falling on the earth at present rates throughout any five billion years of geologic time!
1 Pettersson, op. cit., p. 132,
Similar calculations indicate that enormous quantities of iron would have accumulated on the surface from meteoritic matter during geologic time. Iron is the most abundant element in the meteorites and is also abundant in the earth’s crust.
Can this surface iron be, not the earth’s original substance, but at least in significant part, the accumulated meteoric dust of ages? According to my calculations, the dust would account for all the iron in the upper 11/2 miles of the earth’s solid crust, which certainly accounts, too, for all the iron we’ve managed to dig up.2
2 Isaac Asimov, op. cit., p. 35.
But does anyone actually think that all the iron in the upper l'/2 miles of crust was derived from meteoritic dust? Such a proposition seems out of reason, on its face. Yet this is the strange conclusion to which we are led if meteorite dust has been falling on the earth for anything like five billion years.
It is interesting that radioactivity age calculations made on meteorites are similarly very contradictory.
By examining the helium content of several meteorites, Paneth arrives at ages ranging from 60 million to 7 billion years. . . . Reexamining the evidence, Bauer arrives at a common age of about 60 million years for the meteorites examined by Paneth. This would give us thus a lower limit for the age of the meteorites and also for the age of the universe.1
1 D. Ter Haar: “The Age of the Universe,” Scientific Monthly, Vol. 77, October 1953, p. 177.
It has been difficult for astronomers and geologists to accept such a “small” age for the meteorites in terms of any of the classical theories of the origin of the solar system. More recent and much more subtle calculations have been invoked to “reconcile” the discrepancy.
When that is done, the age of the stony meteorites since solidification is found. The result is about 4.6 thousand million years.2
2G. P. Kuiper, op. cit., p. 15.
Thus, merely by changing the method of calculation, one can increase the age of a meteorite from 60 to 4600 million years! The latter calculation was made by the potassium-argon method, the first by the helium isotope method.
The special type of glassy meteorites known as tektites is still more difficult to interpret. These are found in various localities in the form of what seem to have been showers of the particles.
In contrast to these great ages, the estimated argon ages of tektites (Suess, et al., 1951; Gerling and Yaschenko, 1952) are only one million to ten million years. Gerling and Yaschenko regard this as evidence against a cosmic origin for tektites.3
3 L. H. Ahrens: “Radioactive Methods for Determining Geological Age,” in Physics and Chemistry of the Earth (New York, McGraw-Hill, 1956), p. 60.
Nevertheless, as Stair, in a summary of the evidence, says:
Although some investigators believe that these glass bodies are of terrestrial origin, the preponderance of evidence seems to point to a cosmic source as the origin.4
4 Ralph Stair: “Tektites and the Lost Planet,” Scientific Monthly, Vol. 83, July 1956, p. 4.
The significant feature about the relatively small ages indicated for the tektites is that they seem to be smaller than those of some of the terrestrial strata in which they are deposited.
Each of the major occurrences is thought to be one shower. Those in Czechoslovakia are weathering out of Miocene strata; those in Texas, first described by the writer, are thought to be weathering out of Eocene strata; and those in Australia may be Recent.1
1 Virgil E. Barnes: “Tektites,” Geotimes, Vol. I, No. 12, 1957, p. 6.
Another significant fact, difficult to reconcile with uniformitarianism, is that the tektites are apparently not found in any strata earlier than Tertiary.
Neither tektites nor other meteorites have been found in any of the ancient geological formations.2
2 Ralph Stair, op. cit., p. 11.
This, of course, is hard to reconcile with the generally accepted uniformitarian concept that meteorites have been falling on the earth at essentially present rates throughout some five billion years of geologic time. True meteorites, in fact, have apparently not been found in any but Recent deposits.
It is only the meteorites that escape decomposition in passage through the atmosphere that can possibly be recognized. There probably are many of these, and in the deep sea, where the rate of deposition is extremely slow, cosmic particles may rate high in the sediments as compared to places where other sediments are abundant. No meteorites have ever been found in the geologic column.3
3W. H. Twenhofel: Principles of Sedimentation (2nd Ed., New York, McGrawHill. 1950), p. 144.
The origin and age of comets is even more obscure than that of meteorites. Fred Whipple, who has contributed more to the theory of cometary phenomena than most other modern astronomers, says:
We are still left quite in the dark as to the ultimate origin of comets. Where was the factory in which they were made located, and when did the sun acquire this magnificent assemblage of quite trivial bodies, whose combined total mass, in spite of their vast extent, is probably less than that of the earth?4
4 Fred L. Whipple: "Comets," in The Nov׳ Astronomy (New York, Sinton and Schuster, 1955), p. 207.
The interesting thing about the comets is that they seem to be disintegrating continuously. A number of them have broken up and dissipated within the period of human observation. Evidently all the known comets can be expected to break up and vanish within a time which is geologically very short. Fred Hoyle notes this.
It has been estimated that the break-up of many comets is taking place at such a rate that they will be entirely disrupted within a million years. It is an immediate inference that these comets cannot have been moving around the Sun as they are at present for much longer than a million years, since otherwise they would already have been broken up.1
1Fred Hoyle: Frontiers of Astronomy (Harper and Brothers, New York, 1955), p. 11.
Since comets are very definitely a part of the solar system, the natural inference would be that the maximum age of the comets would also be the maximum age of the solar system, the two having come into existence at approximately the same time. Hoyle avoids this by assuming that the comets did not begin their breaking-up until less than a million years ago! Whipple and most others avoid this conclusion by assuming that there is a gigantic reservoir of “hibernât-ing” comets far out on the edges of the solar gravitational field, almost to the nearest stars. This theory is attributed to Ernst Opik and Jan Oort.
Oort postulated that the cometary cloud may contain as many as 100 billion comets very few of which come as close to the sun as the planets. Occasionally, however, the random passage of a star disturbs the motions of some comets sufficiently to make them swing into the sphere of gravitational attraction of Jupiter or another major planet. In this way comets are taken one by one from the “deep freeze” of the solar swarm and are pulled into relatively short-period orbits. Their hibernation period over, they become active and disintegrate into gas and meteoric particles during a few hundred or few thousand revolutions around the sun.2
2Whipple: op. cil., p. 201-202. See also L. F. Biermann and Rhea List: “The Tails of Comets," Scientific American, Vol. 199, October 1958, p. 44.
There is not the slightest observational basis for this strange theory, nor, as Whipple pointed out, any acceptable theory as to the origin of this hypothetical swarm of hibernating comets. Its only rationale is the need for some escape from the apparent cometary testimony to the youthfulness of the solar system.
Another type of geophysical chronometer indicating an anomalously youthful age of the earth is the accumulation of radioactively derived gases in the atmosphere. The most important of these, of course, is radiogenic helium, which as we have already pointed out, is derived from the disintegration of uranium and thorium in the earth’s crust. Some of this radiogenic helium, of course, escapes and eventually finds its way to the surface, where it is then added to the atmosphere.
But it has been realized for many years that there is not nearly enough helium in the atmosphere to correspond to the supposed age of the earth and the rate of escape of helium from the crustal rocks into the atmosphere.
It may be reasonably supposed that the entire atmospheric supply of Helium-4 is of radioactive origin. Goldschmidt, considering the known helium content of the atmosphere and the known concentrations of uranium and thorium series in primary rocks, concludes that all the atmospheric helium would have been produced in the course of 2 billion years from 2 kilograms per square centimeter of primary rock. This represents about 1.3% of the total amount of primary rock that has been eroded and, which, therefore, might have been expected to have delivered its helium to the atmosphere.1
1 G. E. Hutchinson: “Marginalia,” American Scientist, Vol. 35, January, 1947, p. 118.
This implies that the true maximum age for the earth on the basis of helium production would be only 1.3% of 2 billion, or 26 million years. And even this is impossibly high because it neglects any primary atmospheric helium and any formerly higher radioactive decay rates, as well as helium that may have made its way to the surface from non-denuded rocks.
The method used for avoiding this conclusion is to assume that the excess helium generated in the past has somehow attained the “escape velocity,” overcoming gravity and escaping from the atmosphere completely. This requires that the temperatures in the exosphere (the outermost portion of the atmosphere) must be extremely high.
H. Petersen, F. A. Lindemann and others have shown that the amount of helium released from radio-active rocks during the geological life of the earth exceeds the amount now in the atmosphere. Assuming Stoney’s mechanism to be responsible for the loss of helium that must have occurred, L. Spitzer deduced that the temperature at the critical level is either about 1800 degrees Centigrade or, though usually less, is occasionally more — perhaps for 2 per cent of the time is 2300 degrees Centigrade; and even greater values may be required, for Mayne has recently concluded that the amount of helium released and lost is far greater than has been supposed. Some theorists find the high temperature mentioned difficult to accept.1
1 D. R. Bates: “Composition and Structure of the Atmosphere," in The Earth and Its Atmosphere (New York, Basic Books, Inc., 1957), p. 107.
No independent evidence of such high temperatures yet exists. In other words, instead of accepting the obvious conclusion from the helium content of the atmosphere that the earth’s age must be much less than usually believed, it is rather deduced that the exosphere temperatures must be sufficiently high to permit helium to escape, regardless of how extreme this requirement may be.
Still another evidence of terrestrial youth is found in geochemical analyses of ocean waters. The salts and other chemicals in the sea are being continually augmented, through the process of land denudation and river transportation of the materials of erosion to the sea. Under the assumption that the ocean originally contained none of a specific element and that the rate of supply has always been the same as at present (neither assumption, of course, valid), it is possible to obtain a maximum age for the ocean, and therefore presumably of the earth, on the basis of the measured quantities and rates existing today.
The most common chemicals in ocean water are, of course, sodium and chlorine, the contituents of ordinary table salt, sodium chloride. Sodium averages 10.8 and chlorine 19.6 parts per thousand in ocean water,2 on the average. In average river water these proportions are only 0.0085 and 0.0083 parts per thousand, respectively.3 Oceans constitute about 315,000,000 cubic miles of water volume and rivers about 50,000 cubic miles.1 Of the latter, about 8200 cubic miles annually run off to the seas and are replenished by rainfall. The maximum age of the ocean, as determined from its sodium content is thus computed as
![]() , or about 50 million years. The corresponding chlorine calculation yields about 90 million years. Both are obviously vastly less than 5 billion years!
, or about 50 million years. The corresponding chlorine calculation yields about 90 million years. Both are obviously vastly less than 5 billion years!
2 A. S. Pearse and Gordon Gunter: “Salinity.” Ch. 7 in Treatise on Marine Ecology and Paleoecology, Vol. I, Geological Society of America Memoir 67, 1957, Tables I, II. Sodium and chlorine of course occur in many other compounds in the ocean besides that of sodium chloride.
3 Ibid.
1 Sir Cyril S. Fox: Water (New York, Philosophical Library, 1952), p. xx.
Attempts to make direct estimates of the age of the ocean on the basis of its salt content meet with difficulty. Those based on the amount of sodium in the sea and present rate of erosion put the age at only about fifty million years, a figure that was once accepted as the age of the earth. This figure is only a fraction of that now attributed to the oldest sedimentary rocks, the formation of which depended upon the existence of oceans and continents.2
2 Harold F. Blum: Time’s Arrow and Evolution (Princeton, N. J., Princeton Uni-versily Press, 1951), p, 53.
The usual way of attempting to sidestep this difficulty is to assume a large amount of “cyclic” sodium, etc. — material that has somehow been precipitated on the lands and re-eroded and re-transported, perhaps several times. Of such cyclic sodium there is no definite measure, but even the most generous estimates are inadequate to explain the profound discrepancies.
However it is not thought that the total salt which has been carried into the oceans and has (i) remained there, (ii) been cyclic, (iii) is in rock salt and salt water in the strata can alter the estimate to much more than 200,000,000 years.3
3 Sir Cyril S. Fox, op. cit., p. 27.
This appears to be the maximum figure that can possibly be allowed for the age of the ocean on the basis of its most important chemical constituent.4 But it should be obvious that this is impossibly high because it involves the absurd assumption that the ocean contained no sodium to begin with! Modern marine biologists and oceanographers are, on the other hand, convinced that the salinity of the ocean has always been about as it is now.
4 Other chemicals in the ocean give even shorter age estimates, when calculated on a similar basis. See, for a more extended discussion of this subject, a booklet by D. J. Whitney: How Old Is the Earth? (Malverne, N. Y., Christian Evidence League, n d.). Also, by the same author, The Face of the Deep (New York, Vantage Press, 1955), p. 27-36).
It seems reasonably certain that the salinity of the ocean has remained both quantitatively and qualitatively constant within quite narrow limits since the Cambrian.1
1 G. Evelyn Hutchinson: “Future of Marine Paleoecology,” in Treatise on Marine Ecology and Paleoecology, Vol. II, Geological Society of America Memoir 67, 1957, p. 684.
Indeed there is no reason to doubt that the oceans as great basins of salt water were already present in pre-Cambrian times.2
2 C. S. Fox, loc. cit.
The net result of these considerations would seem to be, quite plainly, that the oceans of the world must be extremely youthful. Both paleo-biological3 and geochemical considerations seem to require that the ocean has always been nearly as saline as at present but that it is continually becoming more saline year by year. This process cannot have been going on for very long.
3 In connection with the salinity of the ocean, a supposed difficulty with the Deluge record has been imagined by some writers, who say that the mixing of salt and fresh waters in a universal Flood would have been fatal to marine creatures accustomed to saline waters and to lacustrine and river fish used to fresh waters. That multitudes of water inhabitants were killed in the Deluge is certain, but there is no reason to suppose the change to have been sudden enough or sharp enough to prevent adaptation of at least some individuals out of each group to their altered environment. The change at the Deluge would, for some time at least, have been to decrease the salinity of most waters and, as Black points out: “Gunter (1942) found that for every fresh-water fish that has been taken in sea water in North America, nine species of marine fish have been taken in fresh water. It seems to be easier for fishes to adapt themselves to excess water than to excess salt” (Vireinia S. Black, in The Physiology of Fishes, New York, Academic Press, 1957, p. 195). An interesting note in Science (Vol. 121, May 27, 1955) describes sharks and sawfish, both marine creatures, found in a fresh water mountain lake 20 miles inland and 500 feet above sea level in western Dutch New Guinea. All fish must be adaptable to at least a certain range of salinities, so it is not unreasonable that some individuals of each kind would be able to survive the gradual mixing of the waters and gradual change in salinities during and after the Flood.
As a matter of fact, there is some basis for believing that the water of the ocean has itself come out of the earth by volcanic emanations in the form of steam and that this process, as well, cannot have continued for a period as long as the supposed age of the lithosphere. It is not ordinarily appreciated what tremendous amounts of juvenile water (that is, water reaching the surface of the earth for the first time) are poured out on the earth’s surface every time a volcano erupts. It is hard to obtain accurate data, of course; probably the best are those that were obtained on the famous Mexican volcano, Paricutin, during the period 1943-52 of its most active life.
If the proportion of water to total solids had been nearly constant throughout the period of activity of the Volcano, the total weight of water expelled would have amounted to some 39 million metric tons — the approximate weight of a body of water about six kilometers square by one meter deep.1
1Carl Fries, Jr.: “Volumes and Weights of Pyroclastic Material, Lava, and Water Erupted by Paricutin Volcano, Michoacan, Mexico,” Transactions, American Geophysical Union, Vol. 34, August 1953, p. 615.
The U.S. Geological Survey personnel making these measurements and studies on Paricutin were of the opinion that all of this water was truly juvenile water. Although there are various theories, most volcanologists now believe this to be true of at least most and probably all of the water expelled from volcanoes.
Until the turn of the present century many geologists considered lava to get its water by seepage from ocean bottoms. Now generally discarded, this view has been replaced by a startling proposal. Volcanic water, say numerous analysts, comes from ‘primary constituents’ — that is, the original matter from which the planet was formed.2
2Gary Webster: “Volcanoes: Nature’s Blast Furnaces,” Science Digest, Vol. 42, November 1957, p. 7.
The Paricutin water described above can be computed as, on the average, about 1/1000 of a cubic mile per year. In view of the fact that there are some 400 or 500 active volcanoes on the continents of the world with several times that number known to have been active in the recent geologic past, we feel it is not unreasonable to guess that the average annual activity of volcanoes in the world has been such as to produce at least one cubic mile of juvenile water each year. Probably this is a gross under-estimate, in view of the tremendous amounts of igneous rocks on and near the surface of the earth which, whatever their method of formation may have been, were certainly accompanied by the expulsion of tremendous amounts of entrapped waters.
It is also known that there are many active volcanoes on the ocean bottom, and there have been many more in the past. Obviously, the number and production of these is almost entirely unknown, but both must be very great. In view of all these factors, we feel that a figure of one cubic mile of water per year, on the average throughout geologic time is a bare minimum estimate of the increment of water added to the ocean.
Since the ocean now contains approximately 315,000,000 cubic miles of water (about 340,000,000 cubic miles if all the water in the earth’s crust and atmosphere, rivers, lakes, etc., is added), a simple calculation1 will yield a figure of 315 to 340 million years as the maximum possible age of the earth, even on the assumption that all the water in the ocean has originated through volcanic action! Once again, this is far less than 4 or 5 billion years.
1 A somewhat similar analysis has been made the basis for the now widely-held opinion among geologists that the ocean has indeed been derived by just this method. See W. W. Rubey: “Geologic History of Sea Water,” Bulletin, Geological Society of America, Vol. 62, pp. llllff. However, for reasons indicated, we believe Rubey and others have grossly over-estimated the time involved.
And of course all this completely ignores the revelation of the initial condition of the created Earth in Genesis 1:2, which describes it as covered with water. Furthermore, it ignores the account of the Deluge, when great volumes of juvenile water were caused to gush forth through the breaking-up of the “fountains of the great deep” and when great volumes of water entered the ocean through the dissipation of the primeval atmospheric vapor blanket.
But, even more amazingly, volcanic action can account for the entire crust of the earth itself in terms of this kind of calculation. That is, if the earth is as old as claimed, emission of volcanic materials at present rates would have produced a volume of material equal to or greater than the volume of rock in all the continents of the world! This is the basis of J. T. Wilson’s remarkable theory that the earth’s crust has developed in just this way.
The emission of lava at the present rate of 0.8 km.3/year throughout the earth’s history of 4.5 x 109 years or even for the 3 x 10’ years since the oldest known rocks were formed would have poured out lava of the order of 3 x 109 km.3 on the Earth’s surface. This corresponds approximately to the volume of the continents (about 30 km. x 1.1 x 10s km.2). A slightly higher rate of volcanism in the early stages of the Earth would allow for the emission of the oceanic crust as well.2
2 J. Tuzo Wilson: “Geophysics and Continental Growth,” American Scientist, Vol. 47, March 1959, p. 14.
Surely the idea that all the rock and soil materials of all the earth’s crust have been built up by volcanic emissions during geologic time is no less strange in terms of traditional uniformitarianism than is the Deluge theory. Although, as we have emphasized, volcanic lavas are of great extent over the earth’s surface, they nevertheless constitute a relatively small proportion of all rocks. Wilson’s supposition is that the granites and other rocks were originally lavas which have since been eroded and metamorphosed from their original condition. This theory is quite speculative, of course, and has not yet attracted any great following. Nevertheless, the arithmetical calculations lead to such a conclusion.
In fact, more realistic calculations would show that the continents could have been derived by volcanic action in much less time than 4.5 billion years. This figure was based on an average lava emission of 0.8 km.3/year. But this latter figure was taken from work by Sapper, which in turn was based on lava flows since 1500 A.D.1 But it is apparent that this rate must be much less than the average rate during geologic time in view of the vastly greater extent of volcanic activity in the past than in the present. Even on the basis of present activity, however, this seems low. The materials (lava and ash) derived from Paricutin during its ten years of activity were over 2000 million cubic meters in volume,2 which therefore averaged 0.2 cubic kilometers per year. Thus, only four such volcanoes would produce Wilson’s 0.8 cubic kilometers per year. If, as we have surmised, the minimum average figure should be at least 1,000 volcanoes, then the above estimate of age would be reduced from 4.5 billion to less than 20 million years. And this on the assumption that all the earth’s crust developed uniformly in this manner!
1 Ibid.
2Fries, op. cit., p. 611.
We have now discussed a number of lines of evidence that seem plainly to show that the estimate of 4 or 5 billion years for the age of the earth must be much too great. Such diversified processes as the fall of meteorites, the break-up of comets, the influx of dissolved chemicals into the ocean, the escape of helium into the atmosphere, the growth of the ocean, and the growth of continents by volcanism, all give ages much less than this. And this is on the basis of the geologist’s own principle of uniformity! Obviously, in terms of the revealed facts of an initial grown Creation and the great discontinuity in all natural processes at the time of the Deluge, even these latter ages must be immensely too large.
Just how much too great is as impossible to determine by scientific calculation as it is to determine the true age of the earth by any of the radioactive minerals. Once again we emphasize that the only certain basis of prehistoric chronology must come by way of divine revelation. This revelation, in the Bible, records a Creation and subsequent universal Flood, both occurring only a few thousand years ago! And nothing in true science can possibly negate this; nor, in fact, when the data are rightly understood, does it even seem to do so.
It may be possible, however, to deduce means of measuring the time since the close of the Deluge phenomena. Except for a period of adjustment to the present normal, it is undoubtedly true that uniform processes have predominated in nature since that time, although we cannot exclude the occasional effects of later lesser catastrophes. This period of adjustment to present rates after the intense activities of the Deluge period does, however, preclude the use of many of these processes for age measurements except for much more recent times, as we have pointed out in the case of the radiocarbon method.
As a matter of fact, men already have at least an approximate chronological framework for post-Deluge history, recorded in the Bible. The traditional Biblical date for the Deluge, as computed in the Ussher chronology, has been about 2350 B.C. (or some 4,300 years ago). There are, of course, various lines of evidence in the Bible itself which militate against the strict-chronology interpretation of the genealogy of Genesis 11:10-26.1 But although the Biblical text does not appear to speak unequivocally as to the date of the Flood, it does give strong witness that this date is on the order of magnitude of only some several thousands of years ago.
1 See Appendix II, pp. 474-489.
And it is very significant that such extra-Biblical information as can be obtained on post-Deluge chronology — whether from archaeological, biological, anthropological, or other sources — all concur in pointing to a time some several millenniums ago from which the present order of things seems to date.
One valuable natural chronometric device is the common tree and its annual growth rings and their patterns. Both living and dead trees can be used in this science, known as dendrochronology, by matching sequences of ring patterns between living trees and beams cut from contemporary trees and these with still earlier beams, etc. The ring patterns are, of course, determined mainly by temperature and precipitation variations from year to year. It might be possible theoretically to extend this chronology back, step by step, using fossil wood, indefinitely. But, as Flint says:
The study of the annual growth rings of trees has yielded a record that extends back through the last 2000-3000 years.1
1 R. F. Flint: Glacial and Pleistocene Geology (New York, Wiley, 1957), p. 292.
More significantly, it is well known that the oldest living things are trees. Many of the giant sequoias are known to be over 3000 years old and, except for unusual catastrophe, seem to be immune to disease and pest attack. A remarkable fact is that these still-living trees seem to be the original trees that grew in their present stands. Note the following very interesting observation:
Perhaps the most intriguing of the unanswered questions regarding longevity in conifers has to do with Sequoia gigantea trees, which, some believe, may enjoy perpetual life in the absence of gross destruction, since they appear immune to pest attack. . . . Pertinent also is the well-known fact that standing snags of this species, other than those resulting from factors of gross destruction, are unknown. Does this mean that shortly preceding 3275 years ago (or 4000 years ago, if John Muir’s somewhat doubtful count was correct) all the then living giant sequoias were wiped out by some catastrophe?2
2Edmund Schulman: “Longevity Under Adversity in Conifers,” Science, Vol. 119, March 26, 1934, p. 399. Of course, no actual evidence of catastrophe was noted, but only the remarkable absence of evidence of any trees of a generation previous to those now growing.
The dendrochronological laboratory at the University of Arizona recently discovered a stand of still older trees in the White Mountains of California, a group of bristlecone pines. Their discoverer says:
Only recently we have learned that certain stunted pines of arid highlands, not the mammoth trees of rainy forests, may now be called the oldest living things on earth.
Microscopic study of growth rings reveals that a bristlecone pine tree found last summer at nearly 10,000 feet began growing more than 4,600 years ago and thus surpasses the oldest known sequoia by many centuries . . . Many of its neighbors are nearly as old; we have now dated 17 bristle-cone pines 4,000 years old or more . . .1
1 Edmund Schulman: “Bristlecone Pine, Oldest Living Thing,” National Geographic Magazine, Vol- 113, March 1958, p. 355.
Since these, as well as the sequoias and other ancient trees, are still living, it is pertinent to ask why these oldest living things apparently have had time to develop only one generation since they acquired their present stands at some time after the Deluge. There is no record of a tree, or any other living thing, being older than any reasonable date for the Deluge.